理论
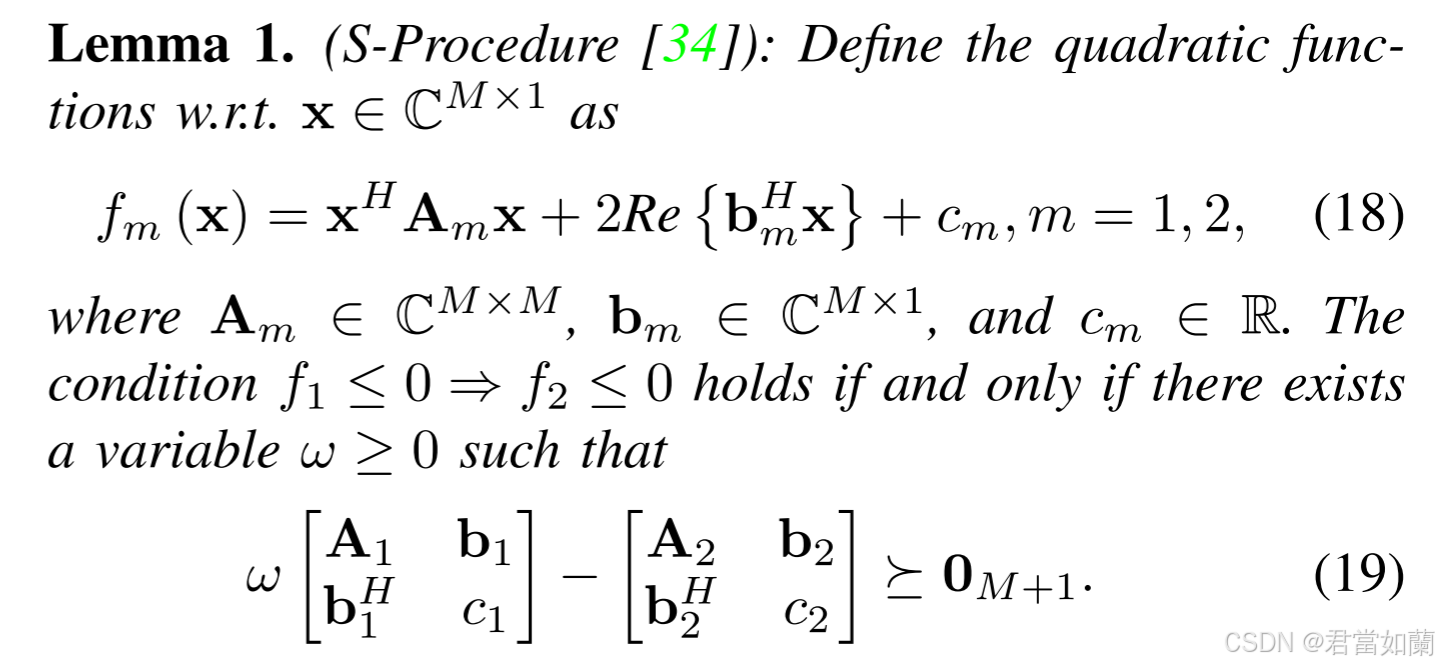
Lemma 1. ( S- Procedure[ 34] ) : Define the quadratic func- \textbf{Lemma 1. ( S- Procedure[ 34] ) : Define the quadratic func- } Lemma 1. ( S- Procedure[ 34] ) : Define the quadratic func-
tions w.r.t. x ∈ C M × 1 \mathbf{x}\in\mathbb{C}^M\times1 x∈CM×1 as
f m ( x ) = x H A m x + 2 R e { b m H x } + c m , m = 1 , 2 , f_m\left(\mathbf{x}\right)=\mathbf{x}^H\mathbf{A}_m\mathbf{x}+2Re\left\{\mathbf{b}_m^H\mathbf{x}\right\}+c_m,m=1,2, fm(x)=xHAmx+2Re{bmHx}+cm,m=1,2,
where A m ∈ C M × M , b m ∈ C M × 1 , a n d \mathbf{A}_m\in\mathbb{C}^{M\times M},\mathbf{b}_m\in\mathbb{C}^{M\times1},and Am∈CM×M,bm∈CM×1,and c m ∈ R . The c_m\in \mathbb{R} . \textit{The} cm∈R.The
c o n d i t i o n f 1 ≤ 0 ⇒ f 2 ≤ 0 h o l d s i f a n d o n l y i f t h e r e e x i s t s condition~f_1\leq0\Rightarrow f_2\leq0~holds~if~and~only~if~there~exists condition f1≤0⇒f2≤0 holds if and only if there exists
a variable ω ≥ 0 such that a\textit{ variable }\omega \geq 0\textit{ such that} a variable ω≥0 such that
(19)
ω [ A 1 b 1 b 1 H c 1 ] − [ A 2 b 2 b 2 H c 2 ] ⪰ 0 M + 1 . \omega\begin{bmatrix}\mathbf{A}_1&\mathbf{b}_1\\\mathbf{b}_1^H&c_1\end{bmatrix}-\begin{bmatrix}\mathbf{A}_2&\mathbf{b}_2\\\mathbf{b}2^H&c_2\end{bmatrix}\succeq\mathbf{0}{M+1}. ω[A1b1Hb1c1]−[A2b2Hb2c2]⪰0M+1.
理论重述
Let f ( x ) f(x) f(x) and g ( x ) g(x) g(x) be two quadratic forms defined as:
f ( x ) = x H A x + 2 ℜ ( b H x ) + c f(x) = x^H A x + 2 \Re(b^H x) + c f(x)=xHAx+2ℜ(bHx)+c
and
g ( x ) = x H D x + 2 ℜ ( e H x ) + f g(x) = x^H D x + 2 \Re(e^H x) + f g(x)=xHDx+2ℜ(eHx)+f
where A , D ∈ C n × n A, D \in \mathbb{C}^{n \times n} A,D∈Cn×n are Hermitian matrices, b , e ∈ C n b, e \in \mathbb{C}^n b,e∈Cn are complex vectors, and c , f ∈ R c, f \in \mathbb{R} c,f∈R are real constants. The superscript H H H denotes the Hermitian (conjugate transpose) of the matrix or vector.
The implication
f ( x ) ≤ 0 ⟹ g ( x ) ≤ 0 f(x) \leq 0 \implies g(x) \leq 0 f(x)≤0⟹g(x)≤0
holds if and only if there exists a scalar λ ≥ 0 \lambda \geq 0 λ≥0 such that:
f ( x ) + λ g ( x ) ≤ 0 f(x) + \lambda g(x) \leq 0 f(x)+λg(x)≤0
or equivalently:
x H ( A + λ D ) x + 2 ℜ ( ( b + λ e ) H x ) + ( c + λ f ) ≤ 0 for all x . x^H (A + \lambda D) x + 2 \Re \left( (b + \lambda e)^H x \right) + (c + \lambda f) \leq 0 \quad \text{for all } x. xH(A+λD)x+2ℜ((b+λe)Hx)+(c+λf)≤0for all x.
This condition can be rewritten as the following matrix inequality:
( A + λ D b + λ e ( b + λ e ) H c + λ f ) ⪰ 0 \begin{pmatrix} A + \lambda D & b + \lambda e \\ (b + \lambda e)^H & c + \lambda f \end{pmatrix} \succeq 0 (A+λD(b+λe)Hb+λec+λf)⪰0
where ⪰ 0 \succeq 0 ⪰0 denotes that the matrix is positive semidefinite (PSD).
Thus, the S-Procedure states that if such a non-negative λ \lambda λ exists, then the implication f ( x ) ≤ 0 ⟹ g ( x ) ≤ 0 f(x) \leq 0 \implies g(x) \leq 0 f(x)≤0⟹g(x)≤0 holds.
实际案例
已知
Δ h H Δ h ≤ a \Delta\mathbf{h}^H \Delta\mathbf{h} \leq a ΔhHΔh≤a
如何根据S-Procedure 理论把下列形式转化成线性矩阵不等式呢
g ( Δ h ) = Δ h H D Δ h + 2 ℜ ( h H D Δ h ) + h H D h − z ≥ 0 g(\Delta\mathbf{h}) = \Delta\mathbf{h}^H \mathbf{D} \Delta\mathbf{h} + 2 \Re(\mathbf{h}^H \mathbf{D} \Delta\mathbf{h}) + \mathbf{h}^H \mathbf{D} \mathbf{h} - z \geq 0 g(Δh)=ΔhHDΔh+2ℜ(hHDΔh)+hHDh−z≥0
实际案例详细说明
\section*{S-Procedure 推导}
\textbf{已知条件}
-
不等式 f 1 ( Δ h ) f_1(\Delta \mathbf{h}) f1(Δh):
f 1 ( Δ h ) = Δ h H Δ h − a ≤ 0 f_1(\Delta \mathbf{h}) = \Delta \mathbf{h}^H \Delta \mathbf{h} - a \leq 0 f1(Δh)=ΔhHΔh−a≤0这表示:
Δ h H Δ h ≤ a \Delta \mathbf{h}^H \Delta \mathbf{h} \leq a ΔhHΔh≤a -
需要证明的不等式 f 2 ( Δ h ) f_2(\Delta \mathbf{h}) f2(Δh):
f 2 ( Δ h ) = − ( Δ h H D Δ h + 2 ℜ ( h H D Δ h ) + h H D h − z ) ≤ 0 f_2(\Delta \mathbf{h}) = - \left( \Delta \mathbf{h}^H \mathbf{D} \Delta \mathbf{h} + 2 \Re (\mathbf{h}^H \mathbf{D} \Delta \mathbf{h}) + \mathbf{h}^H \mathbf{D} \mathbf{h} - z \right) \leq 0 f2(Δh)=−(ΔhHDΔh+2ℜ(hHDΔh)+hHDh−z)≤0等价于:
Δ h H D Δ h + 2 ℜ ( h H D Δ h ) + h H D h − z ≥ 0 \Delta \mathbf{h}^H \mathbf{D} \Delta \mathbf{h} + 2 \Re (\mathbf{h}^H \mathbf{D} \Delta \mathbf{h}) + \mathbf{h}^H \mathbf{D} \mathbf{h} - z \geq 0 ΔhHDΔh+2ℜ(hHDΔh)+hHDh−z≥0
\textbf{应用 S-Procedure}
为了应用 S-Procedure,我们需要构造两个二次型 f 1 f_1 f1 和 f 2 f_2 f2 的矩阵形式,并构造相应的线性矩阵不等式 (LMI)。
-
构造 f 1 ( Δ h ) f_1(\Delta \mathbf{h}) f1(Δh) 的矩阵形式:
f 1 ( Δ h ) = Δ h H Δ h − a f_1(\Delta \mathbf{h}) = \Delta \mathbf{h}^H \Delta \mathbf{h} - a f1(Δh)=ΔhHΔh−a其矩阵形式为:
I 0 0 − a \] \\begin{bmatrix} \\mathbf{I} \& \\mathbf{0} \\\\ \\mathbf{0} \& -a \\end{bmatrix} \[I00−a
-
构造 f 2 ( Δ h ) f_2(\Delta \mathbf{h}) f2(Δh) 的矩阵形式:
f 2 ( Δ h ) = − ( Δ h H D Δ h + 2 ℜ ( h H D Δ h ) + h H D h − z ) f_2(\Delta \mathbf{h}) = - \left( \Delta \mathbf{h}^H \mathbf{D} \Delta \mathbf{h} + 2 \Re (\mathbf{h}^H \mathbf{D} \Delta \mathbf{h}) + \mathbf{h}^H \mathbf{D} \mathbf{h} - z \right) f2(Δh)=−(ΔhHDΔh+2ℜ(hHDΔh)+hHDh−z)可以简化为:
f 2 ( Δ h ) = − Δ h H D Δ h − 2 ℜ ( h H D Δ h ) − ( h H D h − z ) f_2(\Delta \mathbf{h}) = - \Delta \mathbf{h}^H \mathbf{D} \Delta \mathbf{h} - 2 \Re (\mathbf{h}^H \mathbf{D} \Delta \mathbf{h}) - (\mathbf{h}^H \mathbf{D} \mathbf{h} - z) f2(Δh)=−ΔhHDΔh−2ℜ(hHDΔh)−(hHDh−z)其矩阵形式为:
− D − D h − h H D − ( h H D h − z ) \] \\begin{bmatrix} -\\mathbf{D} \& -\\mathbf{D} \\mathbf{h} \\\\ -\\mathbf{h}\^H \\mathbf{D} \& -(\\mathbf{h}\^H \\mathbf{D} \\mathbf{h} - z) \\end{bmatrix} \[−D−hHD−Dh−(hHDh−z)
-
构造 S-Procedure 矩阵:
根据 S-Procedure,存在 μ ≥ 0 \mu \geq 0 μ≥0 使得:
μ [ I 0 0 − a ] − [ − D − D h − h H D − ( h H D h − z ) ] ⪰ 0 \mu \begin{bmatrix} \mathbf{I} & \mathbf{0} \\ \mathbf{0} & -a \end{bmatrix} - \begin{bmatrix} -\mathbf{D} & -\mathbf{D} \mathbf{h} \\ -\mathbf{h}^H \mathbf{D} & -(\mathbf{h}^H \mathbf{D} \mathbf{h} - z) \end{bmatrix} \succeq \mathbf{0} μ[I00−a]−[−D−hHD−Dh−(hHDh−z)]⪰0
进一步简化为:
μ I + D D h h H D − μ a + ( h H D h − z ) \] ⪰ 0 \\begin{bmatrix} \\mu \\mathbf{I} + \\mathbf{D} \& \\mathbf{D} \\mathbf{h} \\\\ \\mathbf{h}\^H \\mathbf{D} \& -\\mu a + (\\mathbf{h}\^H \\mathbf{D} \\mathbf{h} - z) \\end{bmatrix} \\succeq \\mathbf{0} \[μI+DhHDDh−μa+(hHDh−z)\]⪰0