代码随想录Day17 图论-1

DFS和BFS基础

做图论这部分的题目DFS和BFS少不了

DFS是深搜 沿着一条路一直搜索下去直到无法继续向下 再通过回溯 换一条路进行搜索

BFS是广搜 就是从当前节点出发 一直把当前节点所连接的所有节点都搜索过之后 进入下一节点在开始相同的搜索过程

98.所有可达路径

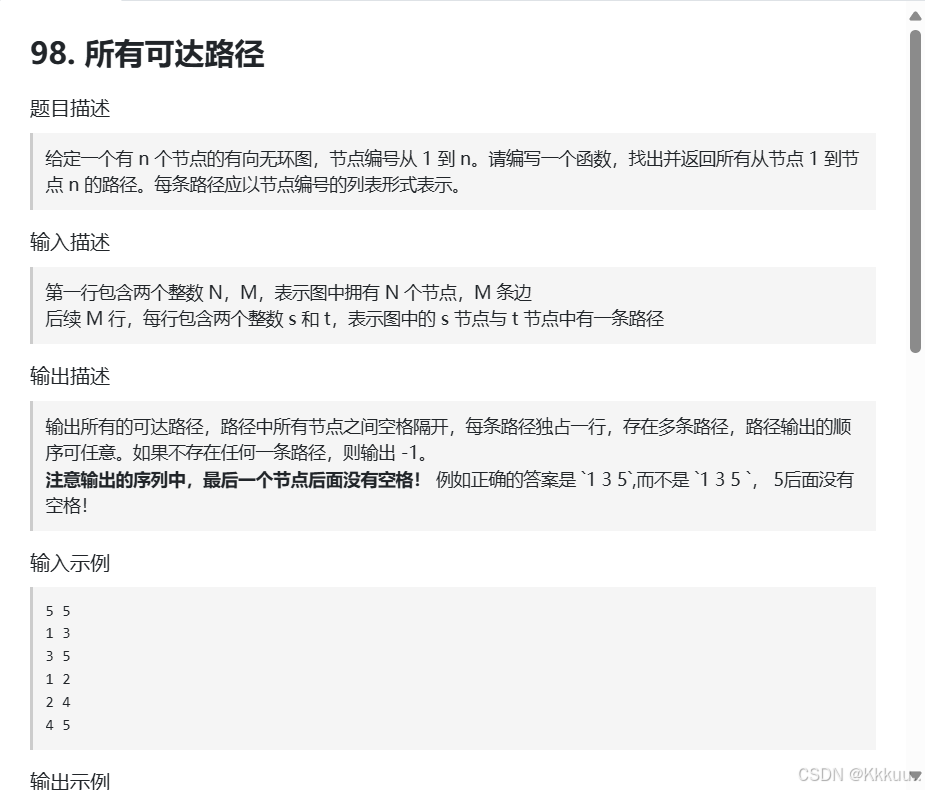
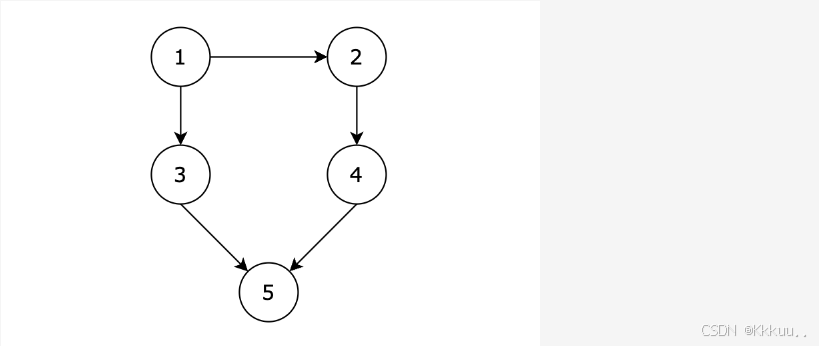
题意很简单 从1节点出发可以到5节点的所有路径 用邻接矩阵构造图 用path记录路径 ans记录所有的路径

dfs 从一条路走到底 遍历当前节点所连接的节点 放入path路径里 然后再进入dfs深搜中 下一个语句就是回溯语句 从path里删除刚刚放入的元素

void dfs(const vector<vector<int>>& graph, int x, int n){
    if(x==n){
        ans.push_back(path);
    }
    for(int i=1;i<=n;i++){
        if(graph[x][i]==1){
            path.push_back(i);
            dfs(graph,i,n);
            path.pop_back();
        }
    }
}

99. 岛屿数量

本题规定了只有上下左右四个方向 相连起来的就是一个岛屿 本题要求存在的岛屿数量

dfs

首先我们需要需要一个dir数组规定四个方向 在主函数里面如果遇到没有访问过的陆地 就让岛屿的总数加1 然后进入dfs

dfs里面逻辑 如果访问的节点访问过或者是水域 就退出dfs 如果是没访问过的陆地就标记为访问过 然后遍历四个方向 用x，y分别记录坐标 判断坐标是否越界 然后进入dfs下一次搜索

int dir[4][2] = {0, 1, 1, 0, -1, 0, 0, -1}; // 四个方向
void dfs(const vector<vector<int>>& grid, vector<vector<bool>>& visited, int x, int y) {
    if (visited[x][y] || grid[x][y] == 0) return; // 终止条件：访问过的节点 或者 遇到海水
    visited[x][y] = true; // 标记访问过
    for (int i = 0; i < 4; i++) {
        int nextx = x + dir[i][0];
        int nexty = y + dir[i][1];
        if (nextx < 0 || nextx >= grid.size() || nexty < 0 || nexty >= grid[0].size()) continue;  // 越界了，直接跳过
        dfs(grid, visited, nextx, nexty);
    }
}

int result = 0;
    for (int i = 0; i < n; i++) {
        for (int j = 0; j < m; j++) {
            if (!visited[i][j] && grid[i][j] == 1) {
                result++; // 遇到没访问过的陆地，+1
                dfs(grid, visited, i, j); // 将与其链接的陆地都标记上 true
            }
        }
    }

bfs

bfs是要把当前节点所连接的节点都要遍历一遍 再进行下一步 所以我们也可以用到队列 只要元素加入队列 我们就标记为访问过 然后当队列不为空的时候 我们取出队列里面的元素 就是当前所遍历的元素 然后遍历四个方向 判断边界是否越界 如果这四个方向的节点有没有访问过的陆地 就把它们加入队列 然后标记

int dir[4][2] = {0, 1, 1, 0, -1, 0, 0, -1}; // 四个方向
void bfs(const vector<vector<int>>& grid, vector<vector<bool>>& visited, int x, int y) {
    queue<pair<int, int>> que;
    que.push({x, y});
    visited[x][y] = true; // 只要加入队列，立刻标记
    while(!que.empty()) {
        pair<int ,int> cur = que.front(); que.pop();
        int curx = cur.first;
        int cury = cur.second;
        for (int i = 0; i < 4; i++) {
            int nextx = curx + dir[i][0];
            int nexty = cury + dir[i][1];
            if (nextx < 0 || nextx >= grid.size() || nexty < 0 || nexty >= grid[0].size()) continue;  // 越界了，直接跳过
            if (!visited[nextx][nexty] && grid[nextx][nexty] == 1) {
                que.push({nextx, nexty});
                visited[nextx][nexty] = true; // 只要加入队列立刻标记
            }
        }
    }
}

100. 岛屿的最大面积

这一题是求所有岛屿中最大的岛屿面积

dfs

我们就用一个count记录当前岛屿的面积 然后每当我们在主函数遇到一个没有访问过的陆地 我们就归零count然后进入dfs深搜 在dfs逻辑里面 标记一个地点就count++

int count;
int dir[4][2] = {0, 1, 1, 0, -1, 0, 0, -1}; // 四个方向
void dfs(vector<vector<int>>& grid, vector<vector<bool>>& visited, int x, int y) {
    if (visited[x][y] || grid[x][y] == 0) return; // 终止条件：访问过的节点 或者 遇到海水
    visited[x][y] = true; // 标记访问过
    count++;
    for (int i = 0; i < 4; i++) {
        int nextx = x + dir[i][0];
        int nexty = y + dir[i][1];
        if (nextx < 0 || nextx >= grid.size() || nexty < 0 || nexty >= grid[0].size()) continue;  // 越界了，直接跳过
        dfs(grid, visited, nextx, nexty);
    }
}

bfs

同dfs一样的思路 代码不一样 创建队列的时候 可以用pair 也可以分别把x，y都放进队列里

int count;
    int dir[4][2] = {0, 1, 1, 0, -1, 0, 0, -1}; // 四个方向
    void bfs(vector<vector<int>>& grid, vector<vector<bool>>& visited, int x, int y) {
        queue<int> que;
        que.push(x);
        que.push(y);
        visited[x][y] = true; // 加入队列就意味节点是陆地可到达的点
        count++;
        while(!que.empty()) {
            int xx = que.front();que.pop();
            int yy = que.front();que.pop();
            for (int i = 0 ;i < 4; i++) {
                int nextx = xx + dir[i][0];
                int nexty = yy + dir[i][1];
                if (nextx < 0 || nextx >= grid.size() || nexty < 0 || nexty >= grid[0].size()) continue; // 越界
                if (!visited[nextx][nexty] && grid[nextx][nexty] == 1) { // 节点没有被访问过且是陆地
                    visited[nextx][nexty] = true;
                    count++;
                    que.push(nextx);
                    que.push(nexty);
                }
            }
        }
    }

101. 孤岛的总面积

本题是求孤岛的总面积 孤岛就是陆地不接触边缘的岛屿 我们可以用一个思路从四个边缘开始搜索 只要遇到陆地就把它变成水域 最后剩下的岛屿就是孤岛

dfs

优化：如果四个方向中有水域就不用继续深搜下去

#include <iostream>
#include <vector>
using namespace std;
int dir[4][2] = {-1, 0, 0, -1, 1, 0, 0, 1}; // 保存四个方向
int count; // 统计符合题目要求的陆地空格数量
void dfs(vector<vector<int>>& grid, int x, int y) {
    grid[x][y] = 0;
    count++;
    for (int i = 0; i < 4; i++) { // 向四个方向遍历
        int nextx = x + dir[i][0];
        int nexty = y + dir[i][1];
        // 超过边界
        if (nextx < 0 || nextx >= grid.size() || nexty < 0 || nexty >= grid[0].size()) continue;
        // 不符合条件，不继续遍历
        if (grid[nextx][nexty] == 0) continue;

        dfs (grid, nextx, nexty);
    }
    return;
}

int main() {
    int n, m;
    cin >> n >> m;
    vector<vector<int>> grid(n, vector<int>(m, 0));
    for (int i = 0; i < n; i++) {
        for (int j = 0; j < m; j++) {
            cin >> grid[i][j];
        }
    }

    // 从左侧边，和右侧边 向中间遍历
    for (int i = 0; i < n; i++) {
        if (grid[i][0] == 1) dfs(grid, i, 0);
        if (grid[i][m - 1] == 1) dfs(grid, i, m - 1);
    }
    // 从上边和下边 向中间遍历
    for (int j = 0; j < m; j++) {
        if (grid[0][j] == 1) dfs(grid, 0, j);
        if (grid[n - 1][j] == 1) dfs(grid, n - 1, j);
    }
    count = 0;
    for (int i = 0; i < n; i++) {
        for (int j = 0; j < m; j++) {
            if (grid[i][j] == 1) dfs(grid, i, j);
        }
    }
    cout << count << endl;
}

bfs

int count = 0;
int dir[4][2] = {0, 1, 1, 0, -1, 0, 0, -1}; // 四个方向
void bfs(vector<vector<int>>& grid, int x, int y) {
    queue<pair<int, int>> que;
    que.push({x, y});
    grid[x][y] = 0; // 只要加入队列，立刻标记
    count++;
    while(!que.empty()) {
        pair<int ,int> cur = que.front(); que.pop();
        int curx = cur.first;
        int cury = cur.second;
        for (int i = 0; i < 4; i++) {
            int nextx = curx + dir[i][0];
            int nexty = cury + dir[i][1];
            if (nextx < 0 || nextx >= grid.size() || nexty < 0 || nexty >= grid[0].size()) continue;  // 越界了，直接跳过
            if (grid[nextx][nexty] == 1) {
                que.push({nextx, nexty});
                count++;
                grid[nextx][nexty] = 0; // 只要加入队列立刻标记
            }
        }
    }
}

102. 沉没孤岛

本题要求我们把孤岛变成0 其实这一题和上一题求孤岛思路差不多 我们同样是先从边缘开始搜索 遇到陆地我们可以赋值为2 然后最后两层for'循环将2赋值成1 将剩下的孤岛1赋值成0

#include <iostream>
#include <vector>
using namespace std;
int dir[4][2] = {-1, 0, 0, -1, 1, 0, 0, 1}; // 保存四个方向
void dfs(vector<vector<int>>& grid, int x, int y) {
    grid[x][y] = 2;
    for (int i = 0; i < 4; i++) { // 向四个方向遍历
        int nextx = x + dir[i][0];
        int nexty = y + dir[i][1];
        // 超过边界
        if (nextx < 0 || nextx >= grid.size() || nexty < 0 || nexty >= grid[0].size()) continue;
        // 不符合条件，不继续遍历
        if (grid[nextx][nexty] == 0 || grid[nextx][nexty] == 2) continue;
        dfs (grid, nextx, nexty);
    }
    return;
}

int main() {
    int n, m;
    cin >> n >> m;
    vector<vector<int>> grid(n, vector<int>(m, 0));
    for (int i = 0; i < n; i++) {
        for (int j = 0; j < m; j++) {
            cin >> grid[i][j];
        }
    }

    // 步骤一：
    // 从左侧边，和右侧边 向中间遍历
    for (int i = 0; i < n; i++) {
        if (grid[i][0] == 1) dfs(grid, i, 0);
        if (grid[i][m - 1] == 1) dfs(grid, i, m - 1);
    }

    // 从上边和下边 向中间遍历
    for (int j = 0; j < m; j++) {
        if (grid[0][j] == 1) dfs(grid, 0, j);
        if (grid[n - 1][j] == 1) dfs(grid, n - 1, j);
    }
    // 步骤二、步骤三
    for (int i = 0; i < n; i++) {
        for (int j = 0; j < m; j++) {
            if (grid[i][j] == 1) grid[i][j] = 0;
            if (grid[i][j] == 2) grid[i][j] = 1;
        }
    }
    for (int i = 0; i < n; i++) {
        for (int j = 0; j < m; j++) {
            cout << grid[i][j] << " ";
        }
        cout << endl;
    }
}