【数据结构】堆

勇闯逆流河2025-05-20 0:37

本文是小编巩固自身而作，如有错误，欢迎指出！

1.树

1.1树的概念与结构

树是⼀种⾮线性的数据结构，它是由 n（n>=0） 个有限结点组成⼀个具有层次关系的集合。把它叫做 树是因为它看起来像⼀棵倒挂的树，也就是说它是根朝上⽽叶朝下的。

树形结构中，子树之间不能有交集，否则就不是树形结构

⾮树形结构：

因此，我们可以得到

• 子树是不相交的

• 除了根结点外，每个结点有且仅有⼀个父结点

• ⼀棵N个结点的树有N-1条边

1.2树的一些 相关术语

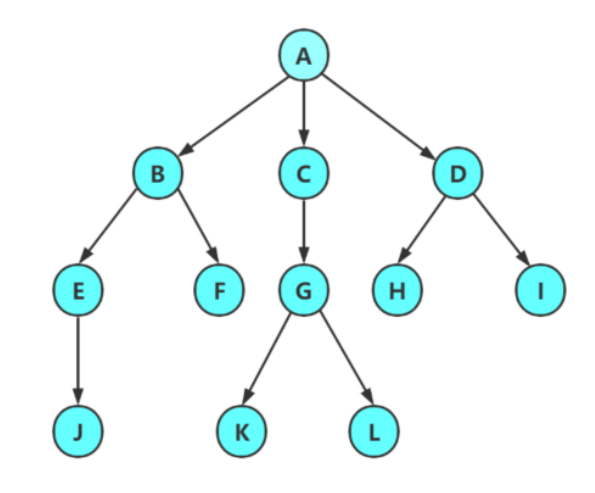
⽗结点/双亲结点：若⼀个结点含有⼦结点，则这个结点称为其⼦结点的⽗结点；如上图：A是B的⽗ 结点

⼦结点/孩⼦结点：⼀个结点含有的⼦树的根结点称为该结点的⼦结点；如上图：B是A的孩⼦结点

结点的度：⼀个结点有⼏个孩⼦，他的度就是多少；⽐如A的度为6，F的度为2，K的度为0 树的度：⼀棵树中，最⼤的结点的度称为树的度；如上图：树的度为 6

叶⼦结点/终端结点：度为 0 的结点称为叶结点；如上图： B、C、H、I... 等结点为叶结点

分⽀结点/⾮终端结点：度不为 0 的结点；如上图： D、E、F、G... 等结点为分⽀结点

兄弟结点：具有相同⽗结点的结点互称为兄弟结点(亲兄弟)；如上图： B、C 是兄弟结

结点的层次：从根开始定义起，根为第 1 层，根的⼦结点为第 2 层，以此类推；

树的⾼度或深度：树中结点的最⼤层次；如上图：树的⾼度为 4

结点的祖先：从根到该结点所经分⽀上的所有结点；如上图： A 是所有结点的祖先

路径：⼀条从树中任意节点出发，沿⽗节点-⼦节点连接，达到任意节点的序列；⽐如A到Q的路径为： A-E-J-Q；H到Q的路径H-D-A-E-J-Q

⼦孙：以某结点为根的⼦树中任⼀结点都称为该结点的⼦孙。如上图：所有结点都是A的⼦孙

森林：由 m（m>0） 棵互不相交的树的集合称为森林；

2.二叉树

2.1二叉树的概念与结构

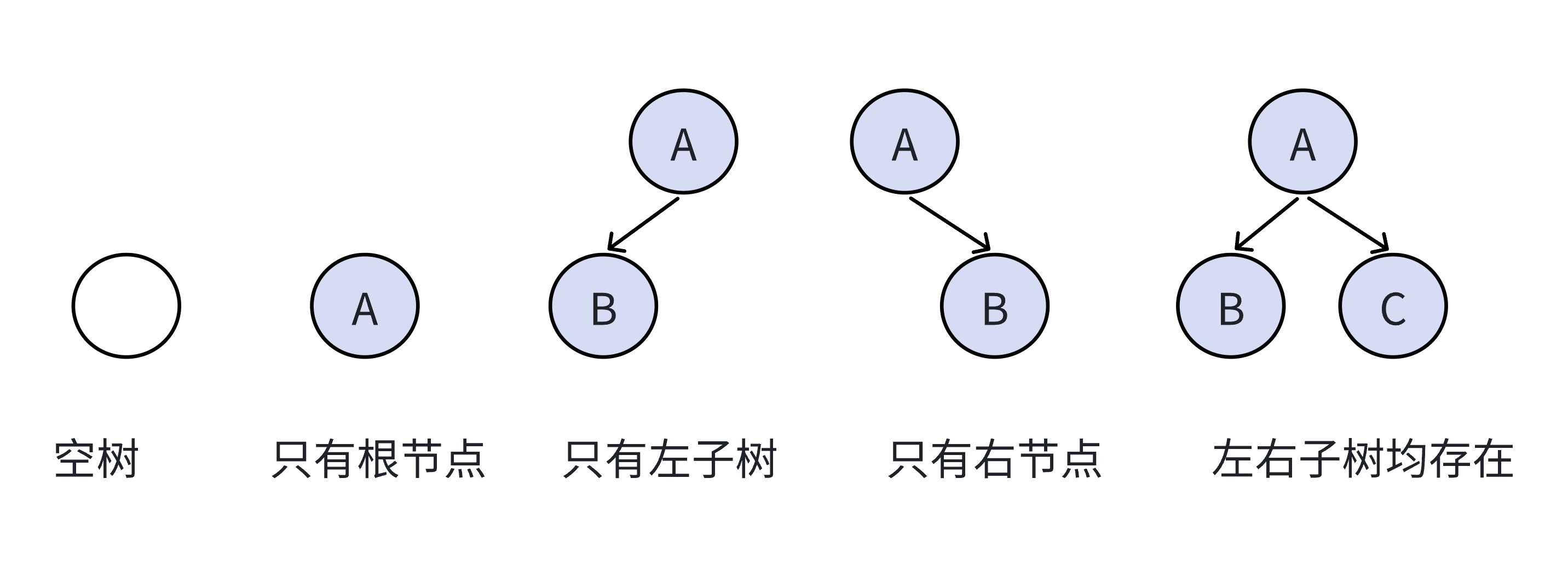
在树形结构中，我们最常⽤的就是⼆叉树，⼀棵⼆叉树是结点的⼀个有限集合，该集合由⼀个根结点 加上两棵别称为左⼦树和右⼦树的⼆叉树组成或者为空。

从上图可以看出⼆叉树具备以下特点：

  1. ⼆叉树不存在度⼤于 2 的结点

  2. ⼆叉树的⼦树有左右之分，次序不能颠倒，因此⼆叉树是有序树

注意：对于任意的⼆叉树都是由以下⼏种情况复合⽽成的

2.2特殊的二叉树

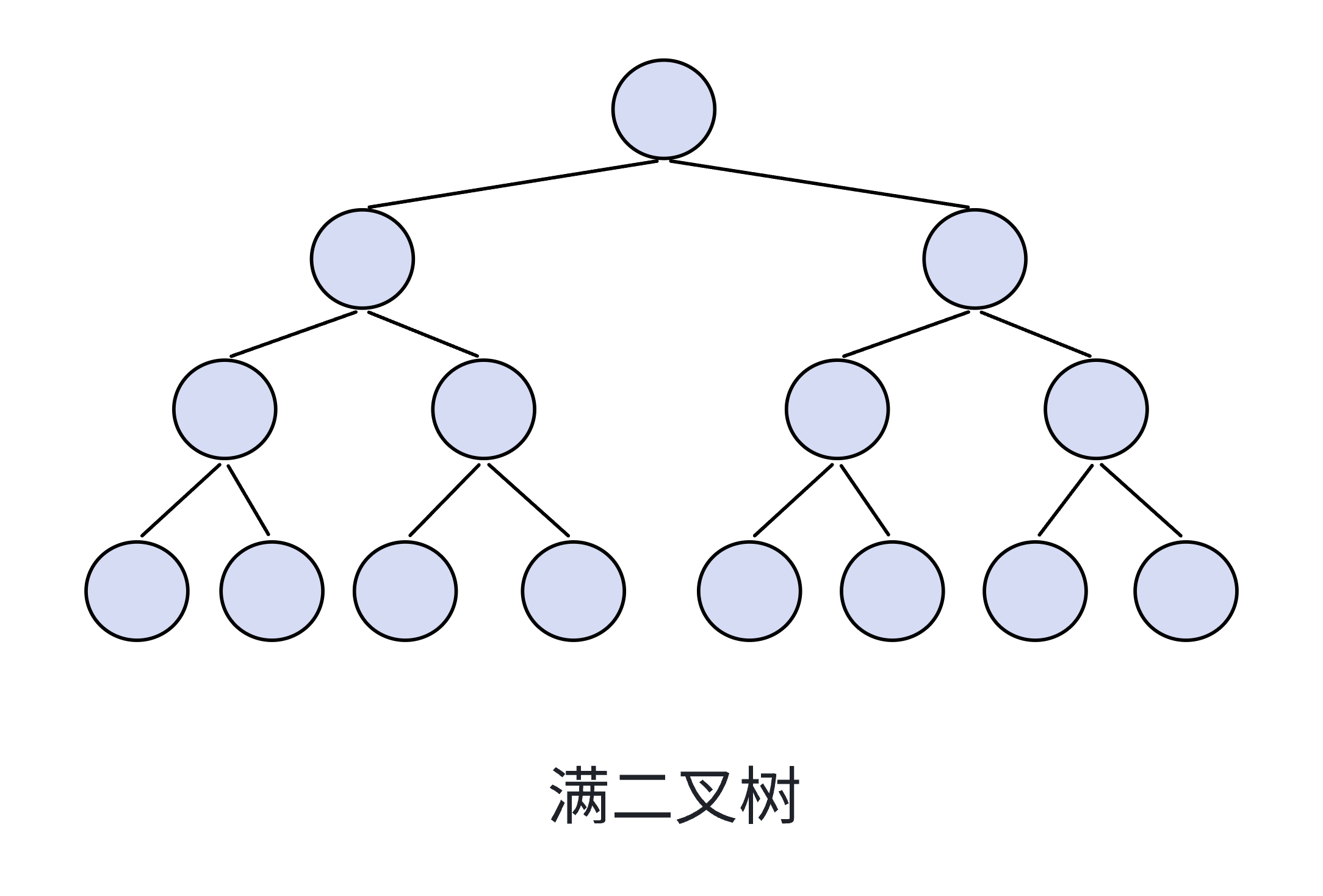
2.2.1满二叉树

⼀个⼆叉树，如果每⼀个层的结点数都达到最⼤值，则这个⼆叉树就是满⼆叉树。也就是说，如果⼀ 个⼆叉树的层数为 K ，且结点总数是2^k −1 ，则它就是满⼆叉树。

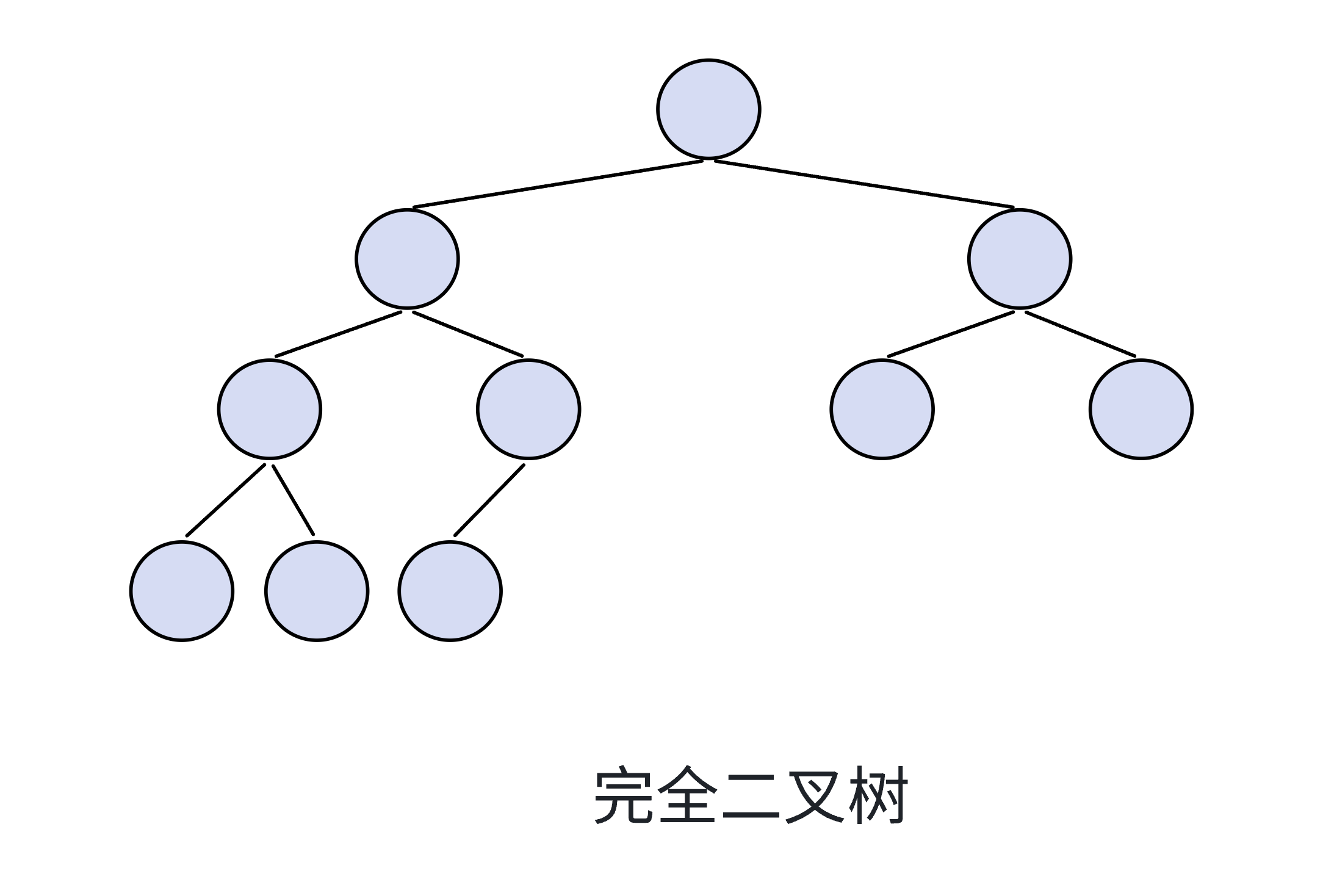
2.2.2完全二叉树

完全⼆叉树是效率很⾼的数据结构，完全⼆叉树是由满⼆叉树⽽引出来的。

对于深度为 K 的，有 n 个 结点的⼆叉树，当且仅当其每⼀个结点都与深度为K的满⼆叉树中编号从 1 ⾄ n 的结点⼀⼀对应时称 之为完全⼆叉树。

要注意的是满⼆叉树是⼀种特殊的完全⼆叉树。

3.堆

⼀般堆使⽤顺序结构的数组来存储数据，堆是⼀种特殊的⼆叉树，具有⼆叉树的特性的同时，还具备 其他的特性。

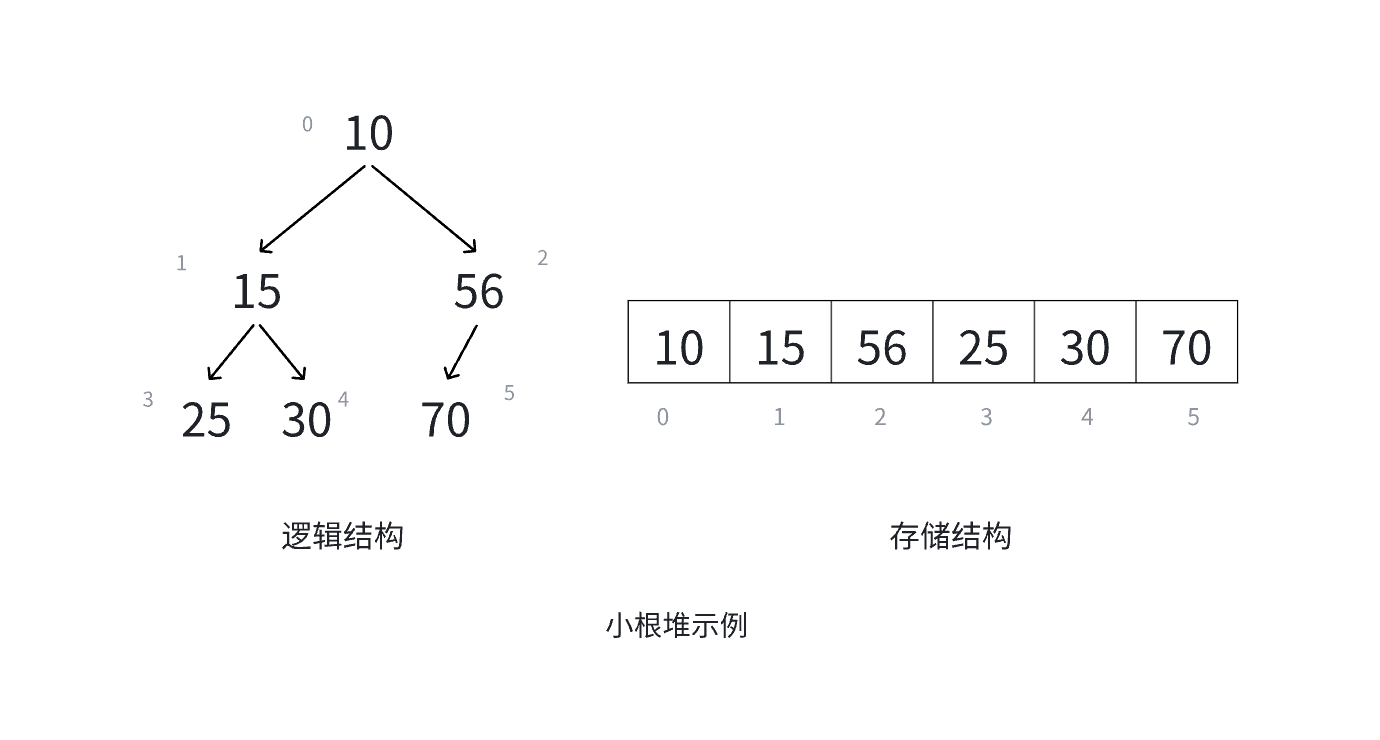
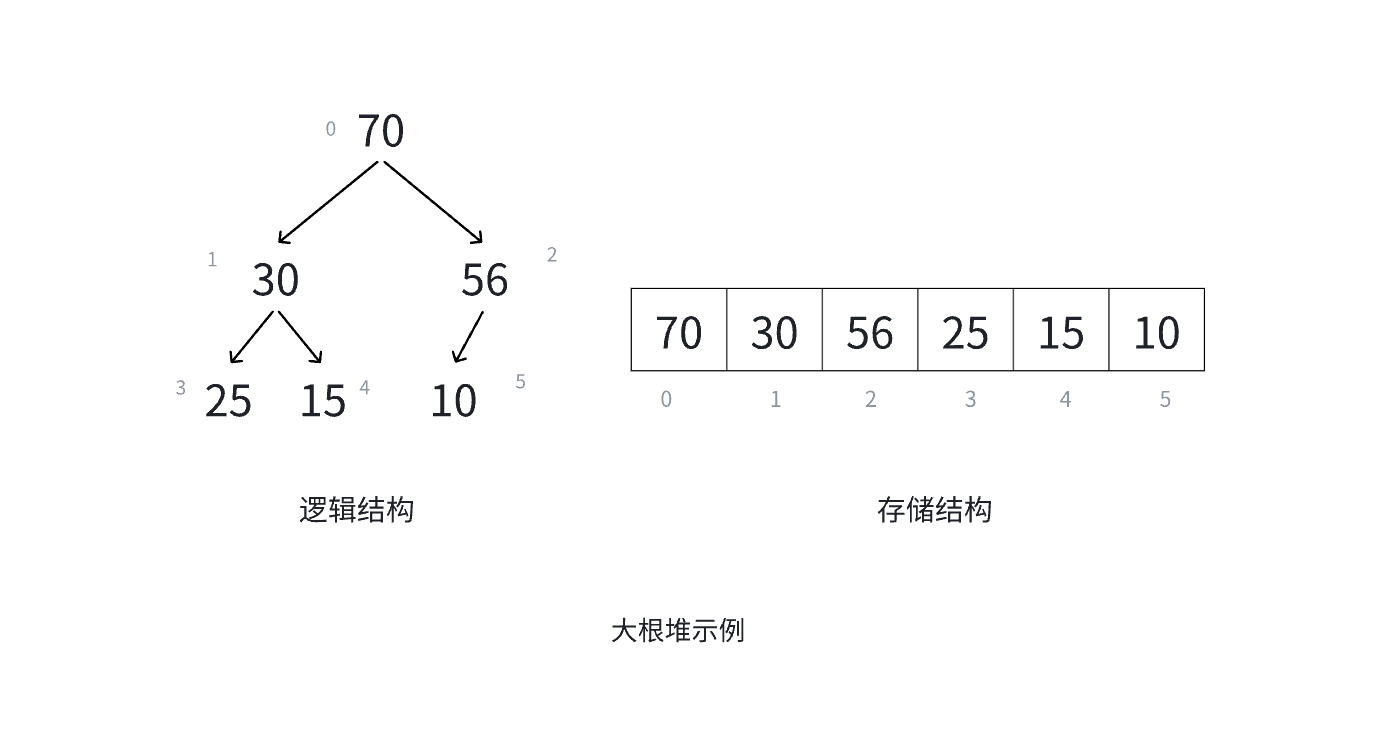
3.1堆的概念与结构

用直白点的话说就是，大数在上面就是大堆，小数在上面就是小堆

二叉树性质

• 对于具有 n 个结点的完全⼆叉树，如果按照从上⾄下从左⾄右的数组顺序对所有结点从 0 开始编号，则对于序号为 i 的结点有：

  1. 若 i>0 ， i 位置结点的双亲序号： (i-1)/2 ； i=0 ， i 为根结点编号，⽆双亲结点

  2. 若 2i+1，左孩⼦序号： 2i+1 ， 2i+1>=n 否则⽆左孩⼦

  3. 若 2i+2，右孩⼦序号： 2i+2 ， 2i+2>=n 否则⽆右孩⼦

3.2堆的实现

3.2.1堆的结构

以数组，类似于顺序表的方式实现

cpp 复制代码 
//堆的结构
typedef int HPdatatype;
typedef struct heap
{
	HPdatatype* arr;
	int size;//有效数据个数
	int capacity;//空间大小

}HP;

3.2.2堆初始化

cpp 复制代码 
void HPinit(HP* php)//堆初始化
{
	php->arr = NULL;
	php->size = php->capacity = 0;
}

3.2.3向堆插入数据

如果是顺序表插入数据，直接将数据尾插即可，可是在堆内插入数据后，就要重新考虑是否要改变顺序，这里我们就采用向上调整算法

向上调整算法的核心思路就是把插入的节点和其母节点进行比较，然后改变顺序。

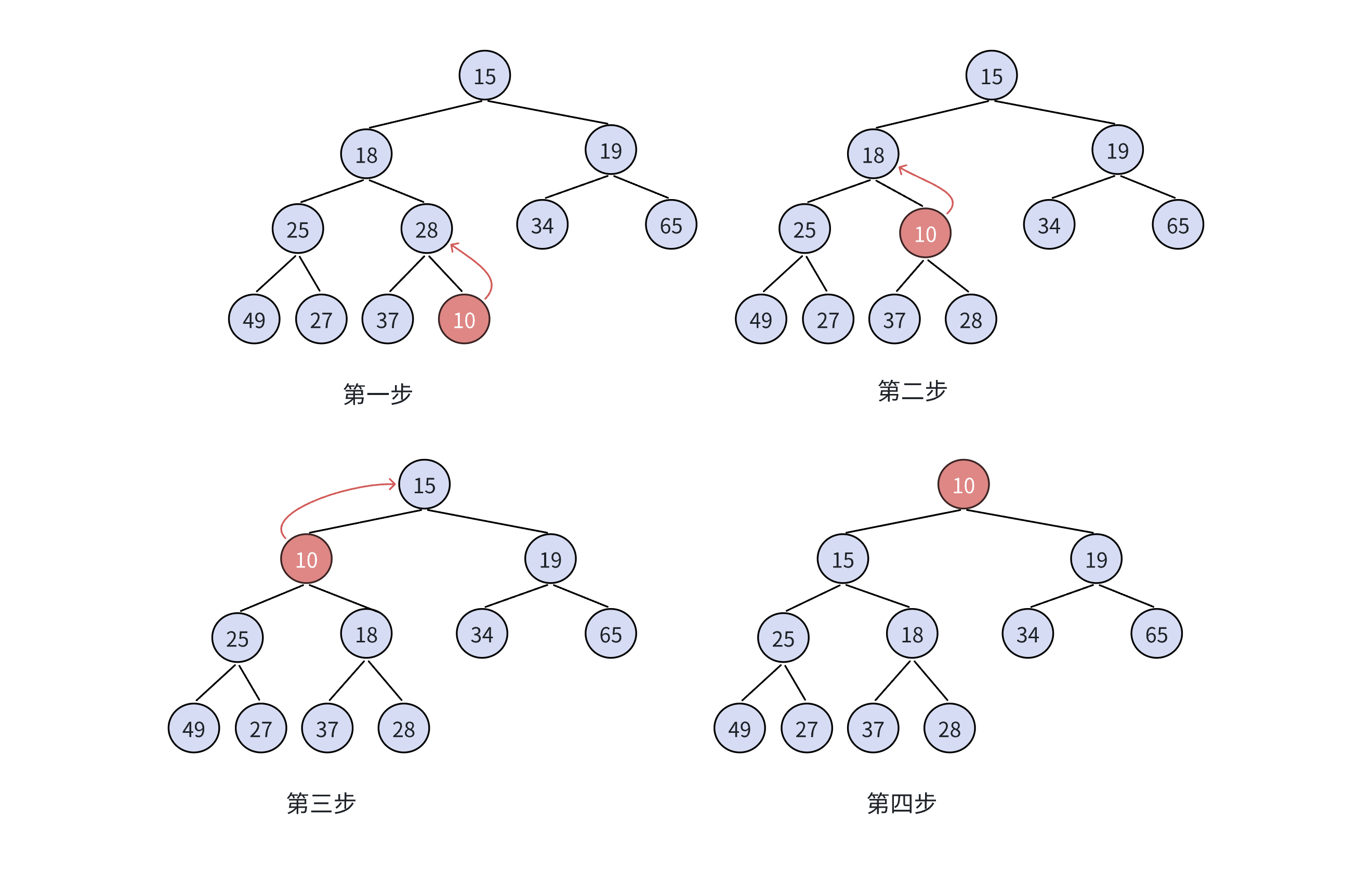
cpp 复制代码 
void Adjustup(HPdatatype* arr,int child)//向上调整算法
{
	int parrent = (child - 1) / 2;//这里的child是指他的下标，不是数据
	while (child > 0)
	{
		if (arr[child] < arr[parrent])
		{
			//调整
			Swap(&arr[child], &arr[parrent]);
			child = parrent;
			parrent = (child - 1) / 2;//母节点和子节点的关系
		}
		else
		{
			break;
		}
	}
}
cpp 复制代码 
void HPpush(HP* php,HPdatatype x)//向堆插入数据
{
	assert(php);
	//判断空间是否足够
	if (php->size == php->capacity)
	{
		int newcapacity = php->capacity == 0 ? 4 : 2 * php->capacity;
		HPdatatype* tmp = (HPdatatype*)realloc(php->arr,sizeof(HPdatatype)*newcapacity);
		if (tmp == NULL)
		{
			perror("realloc fail!");
			exit(1);
		}
		php->arr = tmp;
		php->capacity = newcapacity;
	}
	php->arr[php->size] = x;
	Adjustup(php->arr, php->size);
	php->size++;
	
}

3.2.4出堆

在堆中，出堆是将堆顶的数据删除之后将整个堆重新排序。

在这里我们采用向下调整算法，所谓向下调整算法，就是将最后一个节点和和堆顶交换，然后和子节点进行比较，进行交换。

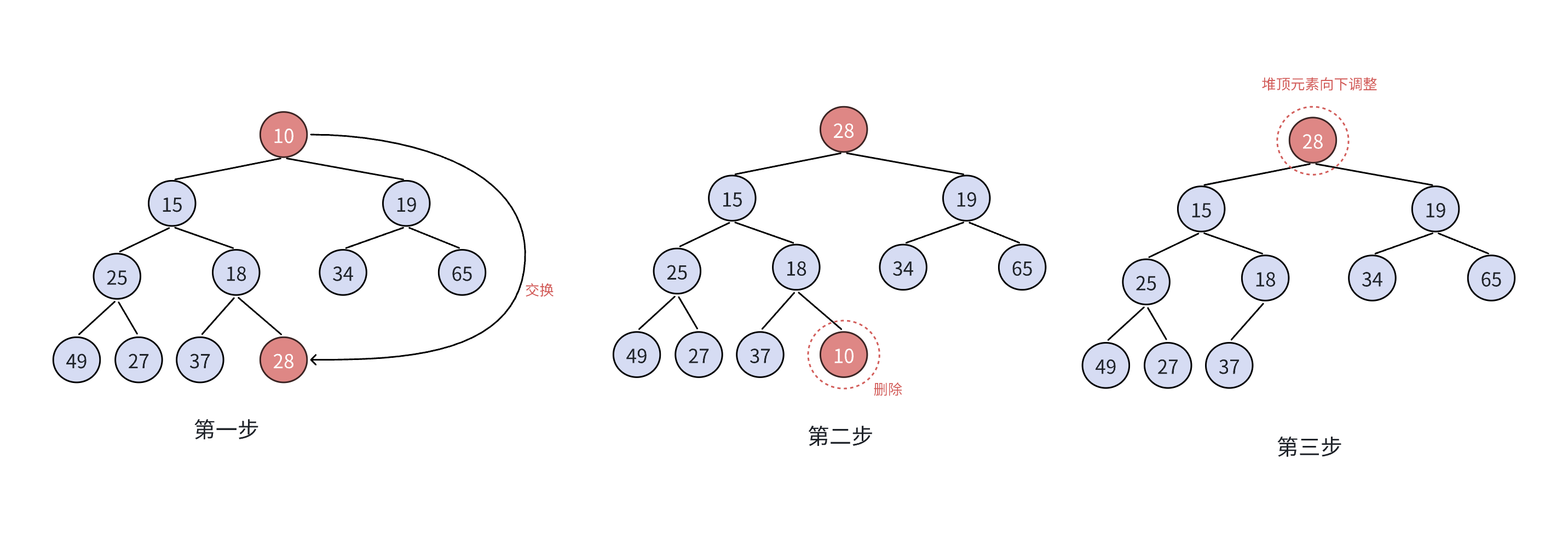
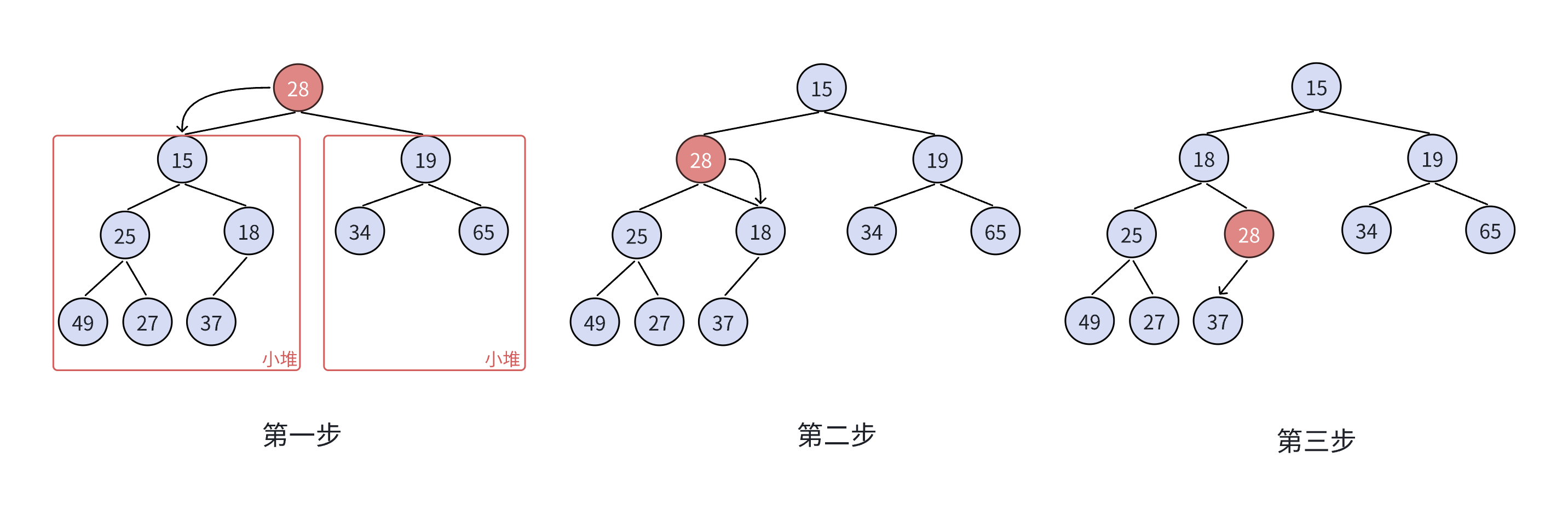
cpp 复制代码 
void Adjustdown(HPdatatype* arr,int parent,int n)//向下调整算法
{
	int child = parent * 2 + 1;//左孩子
	while (child < n)
	{
          //小堆>

		if (child+1<n && arr[child]<arr[child + 1])//确保是将最小（大）的数进行交换	
	    {
			child++;
		}
		//
		if (arr[child] > arr[parent])//小堆<
		{
			//调整
			Swap(&arr[child], &arr[parent]);
			parent = child;
			child = parent * 2 + 1;
		}
		else
		{
			break;
		}
	}
}
cpp 复制代码 
void HPpop(HP* php)//出堆
{
	assert(!HPempty(php));
	//将最开始的父节点删掉
	Swap(&php->arr[0], &php->arr[php->size - 1]);
	--php->size;
	//向下调整
	Adjustdown(php->arr, 0, php->size);

}

3.2.5堆排序

我们根据堆的数据结构，可以较为简单的实现堆排序，如下码

cpp 复制代码 
void HPsort1(int* arr, int n)//堆排序
{
	HP hp;
	HPinit(&hp);
	for (int i = 0;i < n;i++)
	{
		HPpush(&hp, arr[i]);
	}
	int i = 0;
	while (!HPempty(&hp))
	{
		int top = HPtop(&hp);
		arr[i++] = top;
		HPpop(&hp);
	}
	HPdestroy(&hp);
}

但是如果不借助堆的数据结构，该怎么样进行排序呢？

其思路就是：数组建堆，⾸尾交换，交换后的堆尾数据从堆中删掉，将堆顶数据向下调整选出次⼤的数据

cpp 复制代码 
void HPsort2(int* arr, int n)//堆排序
{
	//根据数组建堆，向下调整建堆
	for (int i = (n - 1 - 1) / 2;i > 0;i--)//下标是n-1，父节点是n-1/2
	{
		Adjustdown(arr, i, n);
	}
	//根据堆进行排序
	int end = n - 1;
	while (end > 0)
	{
		Swap(&arr[0], &arr[end]);
		Adjustdown(arr, 0, end);
		end--;
	}

}

其中建堆过程如下

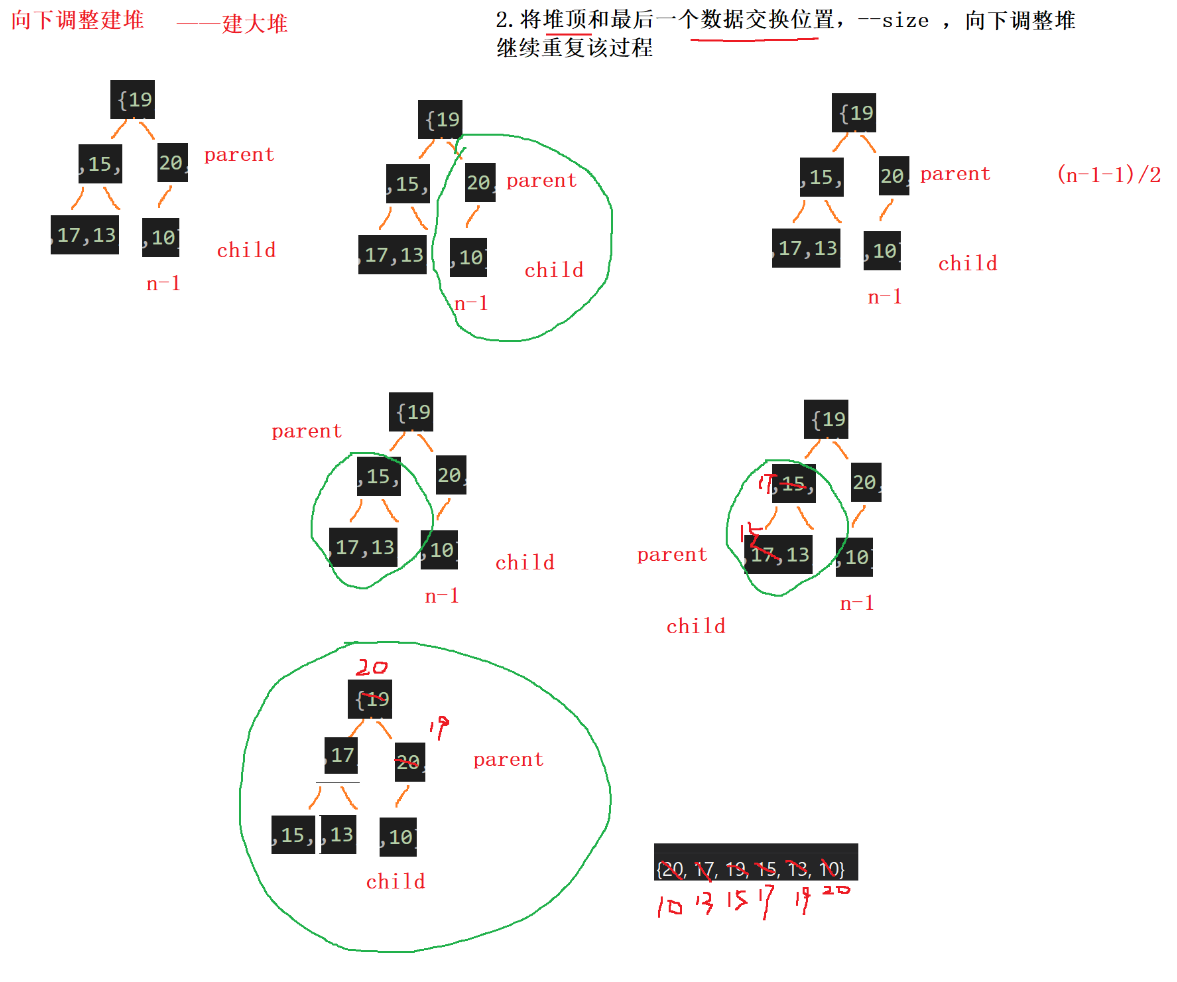
先调整最右子数，再调整相邻的子树。，再调整整个树

然后排序过程的原理就是

将最大和最小的数交换位置，即将最大数储存在数组末端，然后在进行向下调整，此次调整时，将数组的下标自减，就可以最后一个数排除在外，然后重复这个过程，就可以实现排序

3.3完整代码实现

.h文件

cpp 复制代码 
#pragma once
#include<stdio.h>
#include<stdlib.h>
#include<assert.h>
#include<stdbool.h>
//堆的结构
typedef int HPdatatype;
typedef struct heap
{
	HPdatatype* arr;
	int size;//有效数据个数
	int capacity;//空间大小

}HP;

void HPinit(HP* php);//堆初始化
void HPdestroy(HP* php);//销毁堆
void HPpush(HP* php,HPdatatype x);//向堆插入数据
void Adjustup(HPdatatype* arr, int child);//向上调整算法
void Adjustdown(HPdatatype* arr,int parent,int n);//向下调整算法
void Swap(int* x, int* y);//交换函数
void HPprint(HP* php);//打印函数
void HPpop(HP* php);//出堆
bool HPempty(HP* php);//判空
HPdatatype HPtop(HP* php);//取堆顶数据
void HPsort1(int* arr, int n);//堆排序--数据结构
void HPsort2(int* arr, int n);//堆排序

.c文件

cpp 复制代码 
#define _CRT_SECURE_NO_WARNINGS
#include"heap.h"


void HPinit(HP* php)//堆初始化
{
	php->arr = NULL;
	php->size = php->capacity = 0;
}
void HPdestroy(HP* php)//销毁堆
{
	if (php->arr)
	{
		free(php->arr);
		php->arr = NULL;
		php->size = php->capacity = 0;
	}
}
void Swap(int* x, int* y)//交换函数
{
	int tmp = *x;
	*x = *y;
	*y = tmp;
}
void Adjustup(HPdatatype* arr,int child)//向上调整算法
{
	int parrent = (child - 1) / 2;
	while (child > 0)
	{
		//小堆<
	    //大堆>
		if (arr[child] < arr[parrent])
		{
			//调整
			
			Swap(&arr[child], &arr[parrent]);
			child = parrent;
			parrent = (child - 1) / 2;
		}
		else
		{
			break;
		}
	}
}
void HPpush(HP* php,HPdatatype x)//向堆插入数据
{
	assert(php);
	//判断空间是否足够
	
	if (php->size == php->capacity)
	{
		int newcapacity = php->capacity == 0 ? 4 : 2 * php->capacity;
		HPdatatype* tmp = (HPdatatype*)realloc(php->arr,sizeof(HPdatatype)*newcapacity);
		if (tmp == NULL)
		{
			perror("realloc fail!");
			exit(1);
		}
		php->arr = tmp;
		php->capacity = newcapacity;
	}
	php->arr[php->size] = x;
	Adjustup(php->arr, php->size);
	php->size++;
	
}
void HPprint(HP* php)//打印函数
{
	for (int i = 0;i < php->size;i++)
	{
		printf("%d ",php->arr[i]);
	}
	printf("\n");
}
bool HPempty(HP* php)//判空
{
	assert(php);
	return php->size == 0;
}
void Adjustdown(HPdatatype* arr,int parent,int n)//向下调整算法
{
	int child = parent * 2 + 1;//左孩子
	while (child < n)
	{
		if (child+1<n && arr[child]> arr[child + 1])//小堆>
		{
			child++;
		}
		//
		if (arr[child] < arr[parent])//小堆<
		{
			//调整
			Swap(&arr[child], &arr[parent]);
			parent = child;
			child = parent * 2 + 1;
		}
		else
		{
			break;
		}
	}
}
void HPpop(HP* php)//出堆
{
	assert(!HPempty(php));
	//将最开始的父节点删掉
	Swap(&php->arr[0], &php->arr[php->size - 1]);
	--php->size;
	//向下调整
	Adjustdown(php->arr, 0, php->size);

}
HPdatatype HPtop(HP* php)//取堆顶数据
{
	assert(!HPempty(php));
	return php->arr[0];
}
void HPsort1(int* arr, int n)//堆排序
{
	HP hp;
	HPinit(&hp);
	for (int i = 0;i < n;i++)
	{
		HPpush(&hp, arr[i]);
	}
	int i = 0;
	while (!HPempty(&hp))
	{
		int top = HPtop(&hp);
		arr[i++] = top;
		HPpop(&hp);
	}
	HPdestroy(&hp);
}

.c文件（测试）

cpp 复制代码 
#define _CRT_SECURE_NO_WARNINGS
#include"heap.h"

void HPsort2(int* arr, int n)//堆排序
{
	//根据数组建堆，向下调整建堆
	for (int i = (n - 1 - 1) / 2;i >= 0;i--)
	{
		Adjustdown(arr, i, n);
	}
	//根据堆进行排序
	int end = n - 1;
	while (end > 0)
	{
		Swap(&arr[0], &arr[end]);
		Adjustdown(arr, 0, end);
		end--;
	}

}

void test01()
{
	HP hp;
	HPinit(&hp);
	HPpush(&hp, 56);
	HPpush(&hp, 15);
	HPpush(&hp, 10);
	HPpop(&hp);
	HPprint(&hp);
	
	HPdestroy(&hp);
}
test02()
{
	HP hp;
	HPinit(&hp);
	HPpush(&hp, 66);
	HPpush(&hp, 26);
	HPpush(&hp, 76);
	HPprint(&hp);
	printf("\n");
	while (!HPempty(&hp))
	{
		int top = HPtop(&hp);
		printf("%d ", top);
		HPpop(&hp);
	}
	HPdestroy(&hp);
	
}
int main()
{
	//test01();
	/*test02();*/
	int arr[6] = { 19, 23, 45, 43, 22, 11 };
	/*HPsort1(arr, 6);*/
	HPsort2(arr, 6);
	for (int i = 0;i < 6;i++)
	{
		printf("%d ", arr[i]);
	}
	return 0;
}

本次分享就到这里结束了，后续会继续更新，感谢阅读！

相关推荐
pystraf5 小时前
LG P9844 [ICPC 2021 Nanjing R] Paimon Segment Tree Solution
数据结构·c++·算法·线段树·洛谷
飞川撸码6 小时前
【LeetCode 热题100】739：每日温度（详细解析）（Go语言版）
算法·leetcode·golang
yuhao__z6 小时前
代码随想录算法训练营第六十六天| 图论11—卡码网97. 小明逛公园，127. 骑士的攻击
算法
Echo``7 小时前
3：OpenCV—视频播放
图像处理·人工智能·opencv·算法·机器学习·视觉检测·音视频
Nobkins7 小时前
2021ICPC四川省赛个人补题ABDHKLM
开发语言·数据结构·c++·算法·图论
88号技师7 小时前
2025年6月一区SCI-不实野燕麦优化算法Animated Oat Optimization-附Matlab免费代码
开发语言·算法·matlab·优化算法
ysy16480672398 小时前
03算法学习_977、有序数组的平方
学习·算法
codists8 小时前
《算法导论(第4版)》阅读笔记：p83-p85
算法
Tiny番茄9 小时前
归一化函数 ＆ 激活函数
人工智能·算法·机器学习
热门推荐
01从零安装 LLaMA-Factory 微调 Qwen 大模型成功及所有的坑02KGG转MP3工具|非KGM文件|解密音频03YOLOv8入门 | 重要性能衡量指标、训练结果评价及分析及影响mAP的因素【发论文关注的指标】04【SpeedAI科研小助手】2分钟极速解决知网维普重复率、AIGC率过高，一键全文降！文件格式不变，公式都保留的！05组基轨迹建模 GBTM的介绍与实现（Stata 或 R）06Coze扣子平台完整体验和实践（附国内和国际版对比）07Ubuntu24.04安装中文输入法08YOLOv5改进 | 添加CA注意力机制 + 增加预测层 + 更换损失函数之GIoU09DeepSeek各版本说明与优缺点分析10yolov8实战第五天——yolov8+ffmpg实时视频流检测并进行实时推流——（推流，保姆教学）