目录
[P2241 统计方形(数据加强版)](#P2241 统计方形(数据加强版))
[P2089 烤鸡](#P2089 烤鸡)
[P1618 三连击(升级版)](#P1618 三连击(升级版))
[P1036 [NOIP2002 普及组] 选数](#P1036 [NOIP2002 普及组] 选数)
[P1157 组合的输出](#P1157 组合的输出)
[P1706 全排列问题](#P1706 全排列问题)
[P1088 [NOIP2004 普及组] 火星人](#P1088 [NOIP2004 普及组] 火星人)
循环枚举
顾名思义,通过for循环或者while循环枚举所有可能方案。
P2241 统计方形(数据加强版)

很显然这是一道找规律的题目:正方形和长方形的唯一区别在于长宽是否相等,根据此条件可以统计矩形个数,先研究规律:
cppfor (int i = 1; i <= m; i++) for (int j = 1; j <= n; j++)
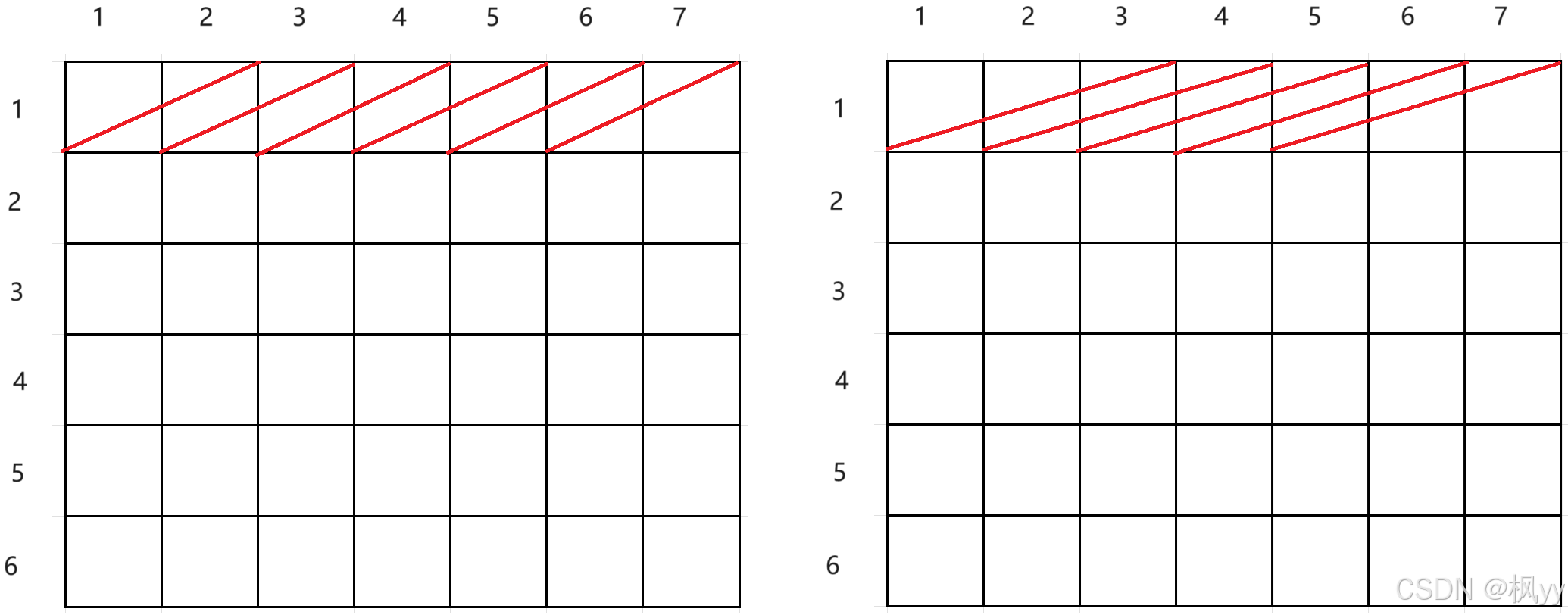
首先是横着的长方形,宽始终为1,长不断发生改变,可以看出长为2的时候,第一行个数为6个,总共有6 x 6个,长为3的时候,总共有6 x 5个......以上述循环条件来看可以得出一个规律:
长发生变化后的矩形总个数为m * ( n - j + 1)个。
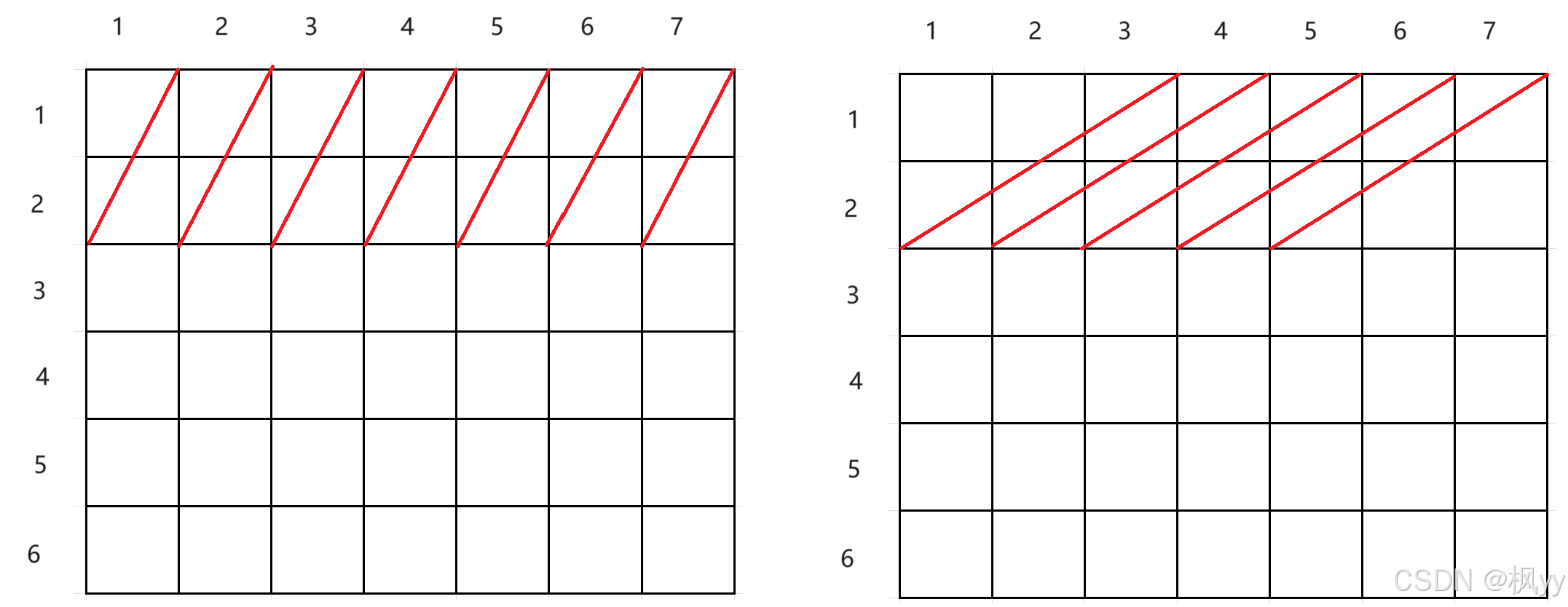
第二看纵向宽发生改变,长重置为1,长为1,宽为2的时候,第一行个数为7个,总共有5 x 7 个,长为2,宽为2的时候 第一行个数为6个,共有5 x 6个......综上所述,可以得出普遍规律:
( m - i + 1) * ( n - j + 1)为每次发生长变化或者宽变化的矩形总个数,又因为长方形与正方形唯一区别是长宽是否相等,因此代码如下:
cpp
#include<iostream>
using namespace std;
int main()
{
int n, m; cin >> n >> m;
long count1 = 0, count2 = 0;
for (int i = 1; i <= m; i++)
for (int j = 1; j <= n; j++)
if (i == j)
count1 += (m - i + 1) * (n - j + 1);
else
count2 += (m - i + 1) * (n - j + 1);
cout << count1 << ' ' << count2 << endl;
return 0;
}P2089 烤鸡

暴力枚举,用十个循环解决此问题,注意:n如果小于10或者大于30直接输出0即可,原因是十种配料之和最小为10,最大为30。
cpp
#include <iostream>
using namespace std;
int main()
{
int n, count = 0; cin >> n;
if (n < 10 || n > 30)
{
cout << 0 << endl;
return 0;
}
else
{
for (int a = 1; a <= 3; a++)
for (int b = 1; b <= 3; b++)
for (int c = 1; c <= 3; c++)
for (int d = 1; d <= 3; d++)
for (int e = 1; e <= 3; e++)
for (int f = 1; f <= 3; f++)
for (int g = 1; g <= 3; g++)
for (int h = 1; h <= 3; h++)
for (int i = 1; i <= 3; i++)
for (int j = 1; j <= 3; j++)
if (a + b + c + d + e + f + g + h + i + j == n)
count++;
cout << count << endl;
for (int a = 1; a <= 3; a++)
for (int b = 1; b <= 3; b++)
for (int c = 1; c <= 3; c++)
for (int d = 1; d <= 3; d++)
for (int e = 1; e <= 3; e++)
for (int f = 1; f <= 3; f++)
for (int g = 1; g <= 3; g++)
for (int h = 1; h <= 3; h++)
for (int i = 1; i <= 3; i++)
for (int j = 1; j <= 3; j++)
if (a + b + c + d + e + f + g + h + i + j == n)
cout << a << ' ' << b << ' ' << c << ' ' << d << ' ' << e << ' ' << f << ' ' << g << ' ' << h << ' ' << i << ' ' << j << ' ' << endl;
}
return 0;
}P1618 三连击(升级版)
本人比较喜欢用stl接口,下面附上代码,注意:输入123,456,789,输出123,456,789
cpp
#include<bits/stdc++.h>
using namespace std;
int a, b, c, t1, t2, t3; string def;
int main()
{
cin >> a >> b >> c;
for (int i = 1; i <= 1000 / c; i++) //记得从1开始 原因:123,456,789满足
{
t1 = i * a; t2 = i * b; t3 = i * c;
string s1 = to_string(t1), s2 = to_string(t2), s3 = to_string(t3);
string tmp; tmp += s1; tmp += s2; tmp += s3;
sort(tmp.begin(), tmp.end()); //排序
auto it = unique(tmp.begin(), tmp.end()); //去重操作
tmp.resize(distance(tmp.begin(), it)); //计算两个迭代器之间的距离
if (tmp.size() == 9 && tmp[0] == '1')
{
cout << s1 << ' ' << s2 << ' ' << s3 << endl;
def = tmp;
}
}
if(def.size()==0) //空的说明都不满足
cout << "No!!!" << endl;
return 0;
}子集枚举
P1036 [NOIP2002 普及组] 选数

这是一道简单的模拟题,枚举出所有可能情况,不会超过规定时间的,以下附上k<=3的代码,如果需要更大的k,继续仿照写即可。
cpp
#include <bits/stdc++.h>
using namespace std;
int n, k;
bool is_prinum(int x)
{
for (int i = 2; i <= sqrt(x); i++)
if (x % i == 0)
return false;
return true;
}
int main()
{
cin >> n >> k;
vector<int> arr(n), pri;
for (int i = 0; i < n; i++)
cin >> arr[i];
int count = 0;
for (int i = 0; i < n; i++)
{
int tmp = arr[i];
if (is_prinum(tmp) && k == 1)
count++;
if (k == 1)
continue;
for (int j = i + 1; j < n; j++)
{
int tmp = arr[i] + arr[j];
if (is_prinum(tmp) && k == 2)
count++;
if (k == 2)
continue;
for (int z = j + 1; z < n; z++)
{
int tmp = arr[i] + arr[j] + arr[z];
if (is_prinum(tmp) && k == 3)
count++;
if (k == 3)
continue;
}
}
}
cout << count << endl;
return 0;
}P1157 组合的输出
与上面一题类似,也是求子集,直接for循环叠加:,下面只举例到3:
cpp
#include <bits/stdc++.h>
using namespace std;
int n, k;
int main()
{
cin >> n >> k;
vector<string> arr(n), ans;
for (int i = 0; i < n; i++)
arr[i] = to_string(i + 1);
for (int i = 0; i < n; i++)
{
if (k == 1)
{
cout << setw(3) << stoi(arr[i]) << endl;
continue;
}
for (int j = i + 1; j < n; j++)
{
if (k == 2)
{
cout << setw(3) << arr[i] << setw(3) << arr[j] << endl;
continue;
}
for (int z = j + 1; z < n; z++)
{
if (k == 3)
{
cout << setw(3) << arr[i] << setw(3) << arr[j] << setw(3) << arr[z] << endl;
continue;
}
}
}
}
return 0;
}排列枚举
P1706 全排列问题
本题可以点击此链接看我另一篇文章,其中解释了如何使用stl库的函数解决该问题。
P1088 [NOIP2004 普及组] 火星人
本题不过多赘述,与上题一样也是stl的使用,以下为代码:
cpp
#include<bits/stdc++.h>
using namespace std;
int main()
{
int n, m; cin >> n >> m;
vector<int> arr(n);
for (int i = 0; i < n; i++)
cin >> arr[i];
for (int j = 1; j <= m; j++)
next_permutation(arr.begin(), arr.end());
for (auto e : arr)
cout << e << ' ';
return 0;
}