C++ 二叉树进阶：二叉搜索树

目录

二叉搜索树的概念

二叉搜索树的实现

基本结构

插入

1，当树是空树的时候

2，当树不为空的时候

3，纠正后的代码

查找

删除

1，左为空或右为空

2，左右都不为空

3，删除的完整代码：

二叉搜索树的完整代码

BSTree.h

test.cpp

二叉搜索树的应用

[Key 模型](#Key 模型)

[Key-Value 模型](#Key-Value 模型)

改造二叉搜索树为KV结构

BSTree.h

test.cpp

二叉搜索树的性能分析

---

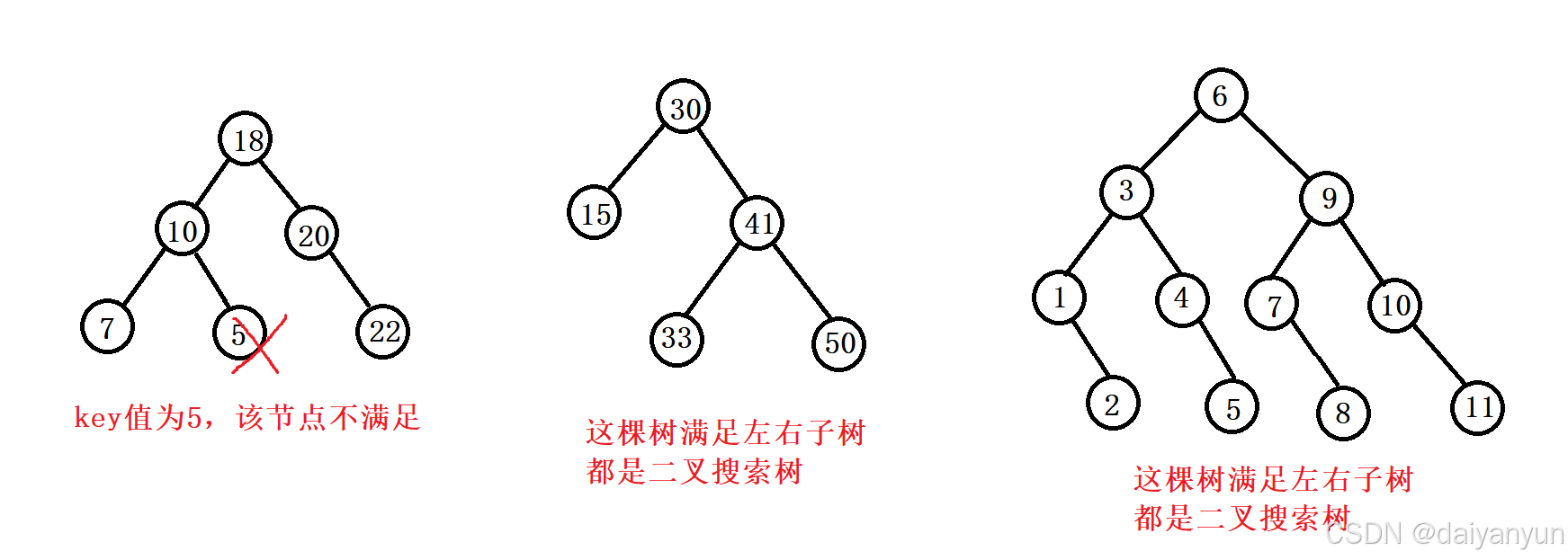
二叉搜索树的概念

二叉搜索树又称二叉排序树，它或者是一棵空树，或者是具有以下性质的二叉树:

若它的左子树不为空，则左子树上所有节点的值都小于根节点的值

若它的右子树不为空，则右子树上所有节点的值都大于根节点的值

它的左右子树也分别为二叉搜索树

注意：二叉搜索树key值不能相同。

二叉搜索树中序遍历是有序的，因为二叉搜索树的定义决定了左子树节点值小于根节点值、右子树节点值大于等于根节点值(每一颗子树也满足)，而中序遍历先左子树、再根节点、后右子树的方式使得遍历结果自然有序。

---

二叉搜索树的实现

基本结构

二叉搜索树中的每个节点包含两个指针，分别指向左子树和右子树，以及一个存储关键值（key 值）的数据域。这种结构使得二叉搜索树能够以二叉树的形式组织数据，并通过比较节点的关键值来进行高效的查找、插入和删除操作。

二叉搜索树不能修改里面的key值，如果修改了就会破坏二叉搜索树的结构。

//节点的定义
template<class K>
struct BSTreeNode
{
	BSTreeNode<K>* _left;   //左节点
	BSTreeNode<K>* _right;  //右节点
	K _key;      //存储 key 值
	BSTreeNode(const K& key)  //构造函数完成初始化
		:_left(nullptr)
		,_right(nullptr)
		,_key(key)
	{}
};

template <class K>  //key 关键字，进行比较
class BSTree  //Binary Search Tree
{
	typedef BSTreeNode<K> Node;
private:
	Node* _root = nullptr;  //在类内进行成员初始化
};

插入

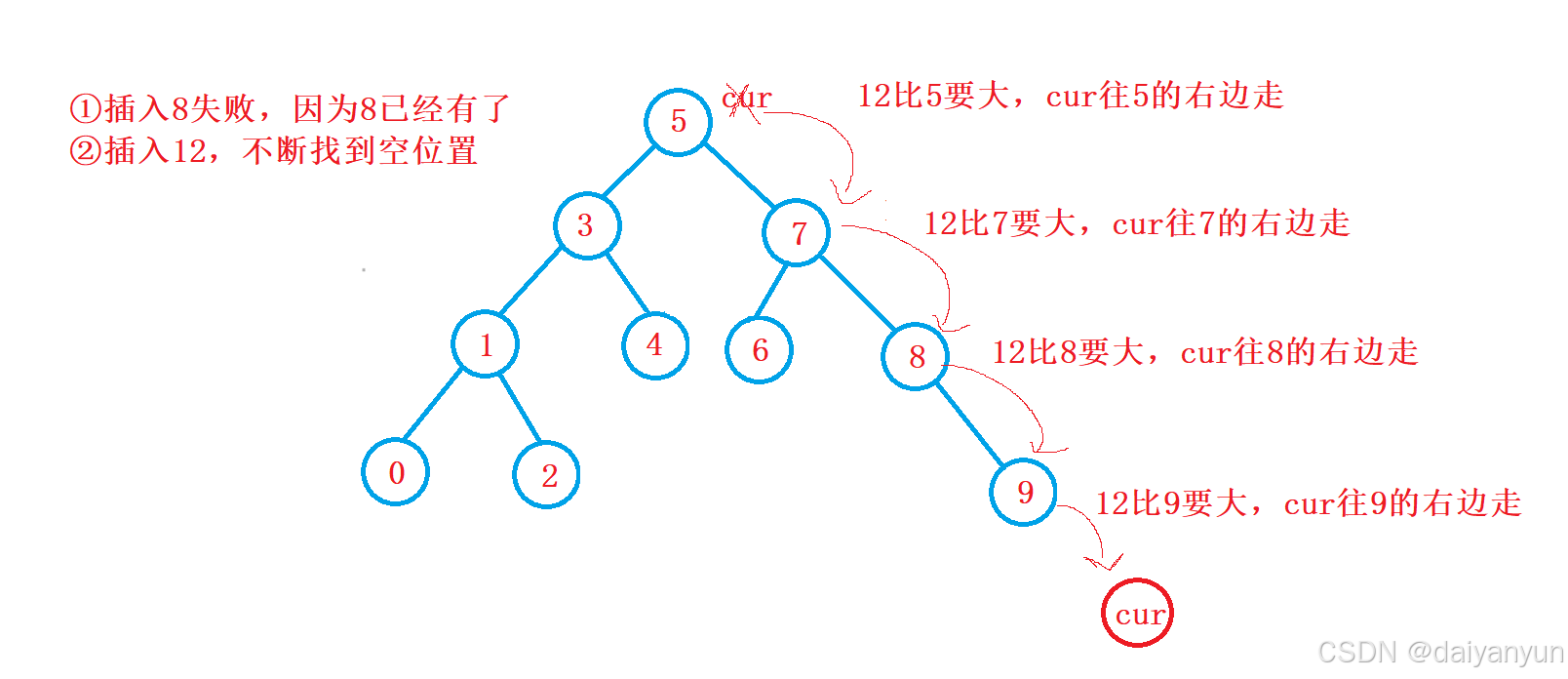
1，当树是空树的时候

直接定义一个节点把该节点给 _root。

2，当树不为空的时候

不是空树，去找这个需要插入的位置，插入一定是找一个空的位置，不可能替代某个位置。

如果插入时是相同的元素，则插入失败，因为二叉搜索树不允许出现相同的 key 值。

#pragma once
//节点的定义
template<class K>
struct BSTreeNode
{
	BSTreeNode<K>* _left;   //左节点
	BSTreeNode<K>* _right;  //右节点
	K _key;      //存储 key 值
	BSTreeNode(const K& key)  //构造函数完成初始化
		:_left(nullptr)
		,_right(nullptr)
		,_key(key)
	{}
};

template <class K>  //key 关键字，进行比较
class BSTree  //Binary Search Tree
{
	typedef BSTreeNode<K> Node;
public:
	bool Insert(const K& key)
	{
		//1，根为空的时候
		if (_root == nullptr)
		{
			_root = new Node(key);
		}
		//2,根不为空的时候
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key)   //插入的key比当前节点大就往右边走
			{
				cur = cur->_right;   
			}
			else if (key < cur->_key) //插入的key比当前节点小就往左边走
			{
				cur = cur->_left;
			}
			else                     
			{
				return false; //插入的key和当前节点相等，就插入失败
			}
		}
		cur = new Node(key);
		return true;
	}
	//中序遍历
	void _InOrder(Node* root) 
	{
		if (root == nullptr)
			return;
		_InOrder(root->_left);
		cout << root->_key << " ";
		_InOrder(root->_right);
	}
	void InOrder() 
	{
		_InOrder(_root);
		cout << endl;
	}
private:
	Node* _root = nullptr;  //在类内进行成员初始化
};
void Test() 
{
	BSTree<int> t;
	int a[] = { 5,3,4,1,7,8,2,6,0,9 };
	for (auto e : a)
	{
		t.Insert(e);
	}
	t.InOrder();  
}

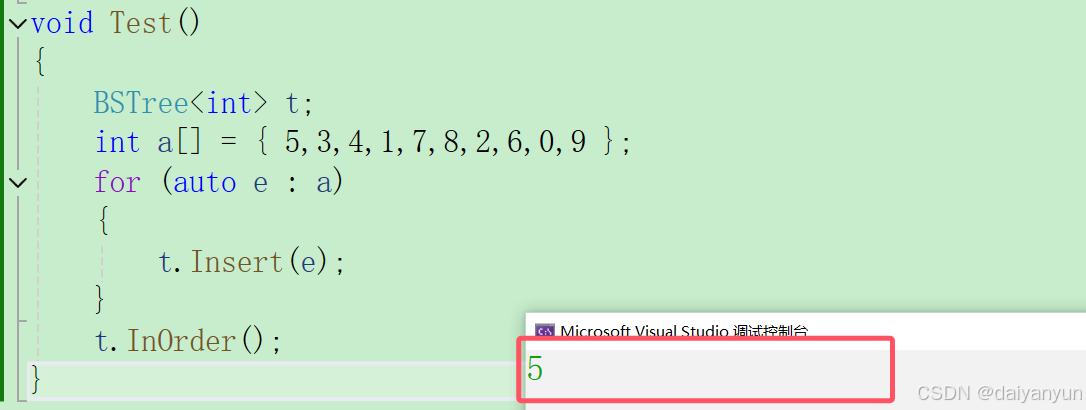
通过测试我们会发现，这里只有 5 插入成功了，也就是根节点插入成功，那么这段代码存在一定的问题，如何解决呢？？？

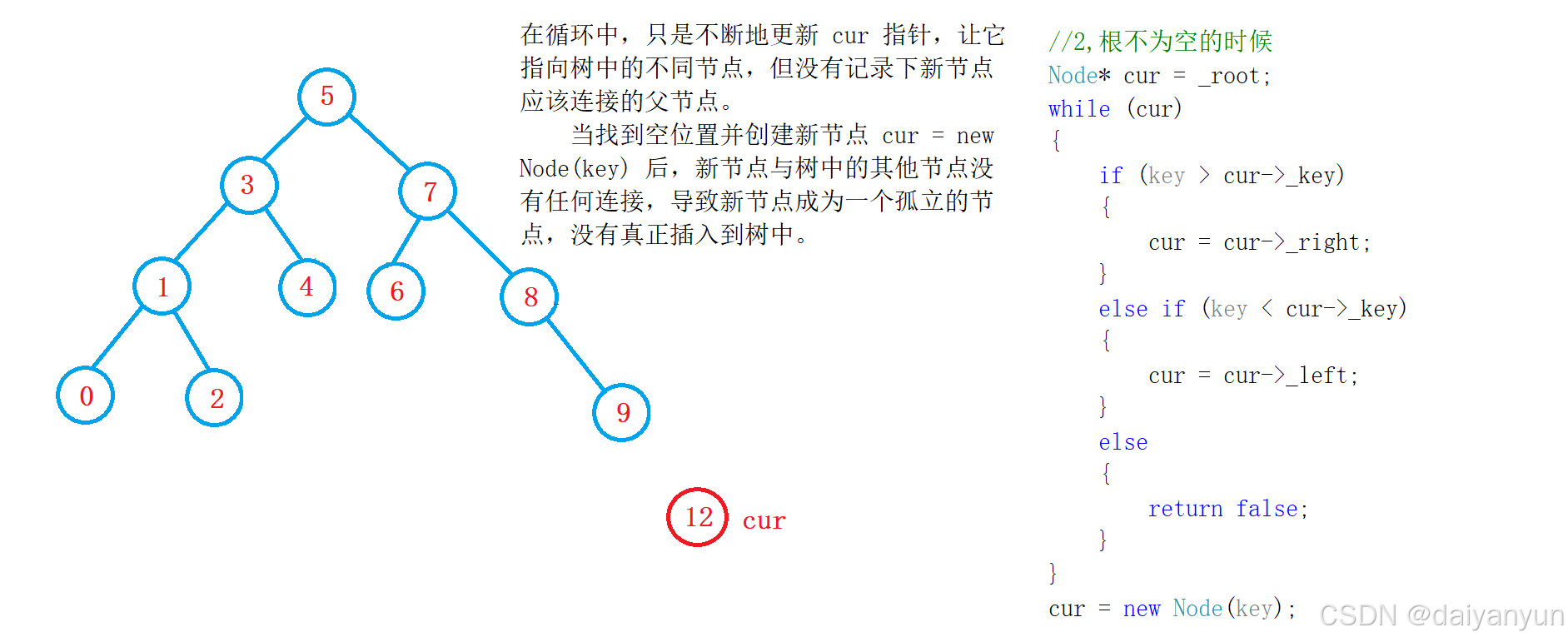
问题： 在循环中，只是不断地更新 cur 指针，让它指向树中的不同节点，但没有记录下新节点应该连接的父节点。

当找到空位置并创建新节点 cur = new Node(key) 后，新节点与树中的其他节点没有任何连接，导致新节点成为一个孤立的节点，没有真正插入到树中。

改进：

1. 添加一个 parent 指针来记录新节点的父节点。在循环中，当更新 cur 指针时，也同时更新 parent 指针。
2. 在找到插入位置后，根据 key 与 parent->_key 的大小关系，将新节点连接到父节点的左子树或右子树。

3，纠正后的代码

#pragma once
//节点的定义
template<class K>
struct BSTreeNode
{
	BSTreeNode<K>* _left;   //左节点
	BSTreeNode<K>* _right;  //右节点
	K _key;      //存储 key 值
	BSTreeNode(const K& key)  //构造函数完成初始化
		:_left(nullptr)
		,_right(nullptr)
		,_key(key)
	{}
};

template <class K>  //key 关键字，进行比较
class BSTree  //Binary Search Tree
{
	typedef BSTreeNode<K> Node;
public:
	bool Insert(const K& key)
	{
		//当树是空树的时候
		if (_root == nullptr) 
		{
			_root = new Node(key);
			return true;
		}
		//树不为空的时候
		Node* parent = nullptr;  //用一个节点来记录cur的父亲
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key) 
			{
				parent = cur;   
				cur = cur->_right;	
			}
			else if (key < cur->_key) 
			{
				parent = cur;
				cur = cur->_left;
			}
			else
			{
				return false;
			}
		}
		cur = new Node(key);
		if (key > parent->_key)  //判断到底是属于父亲的左树还是右树
			parent->_right = cur;
		else
			parent->_left = cur;
		return true;
	}

	//中序遍历
	void _InOrder(Node* root) 
	{
		if (root == nullptr)
			return;
		_InOrder(root->_left);
		cout << root->_key << " ";
		_InOrder(root->_right);
	}
	void InOrder() 
	{
		_InOrder(_root);
		cout << endl;
	}
private:
	Node* _root = nullptr;  //在类内进行成员初始化
};
void Test() 
{
	BSTree<int> t;
	int a[] = { 5,3,4,1,7,8,2,6,0,9 };
	for (auto e : a)
	{
		t.Insert(e);
	}
	t.InOrder();  
}

查找

查找和插入类似，如果比当前节点小就往左边找，如何比当前节点大就往右边找，不断更新cur，直到找返回 true，如果没有找到，返回 false。

	//查找
	bool Find(const K& key) 
	{
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key) 
			{
				cur = cur->_right;
			}
			else if (key < cur->_key) 
			{
				cur = cur->_left;
			}
			else 
			{
				return true;
			}
		}
		return false;
	}

删除

二叉搜索树重点在于删除操作，也比较简单。

在实现Erase的时候我们不能使用Find，因为我们还需用到它的父亲，所以这里还是使用双指针。

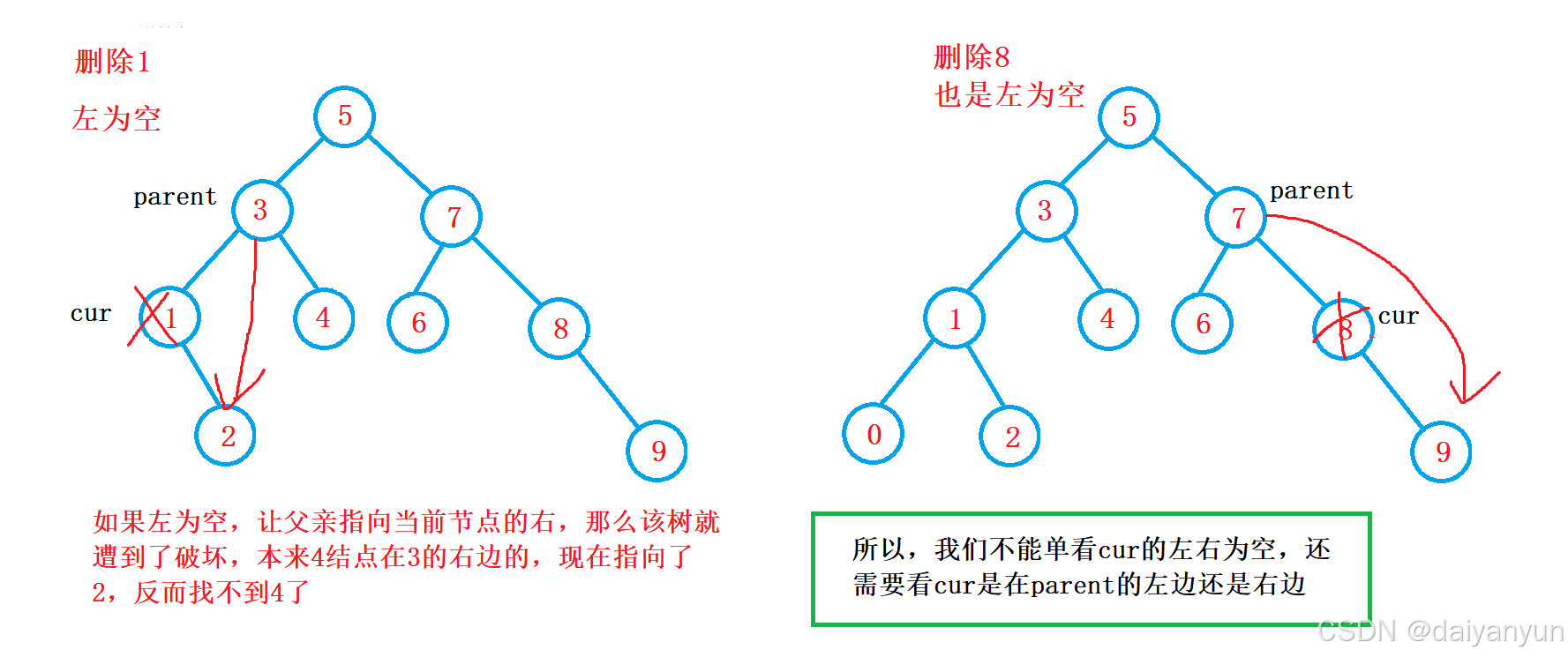
当我们试删除这些节点，我们可以发现可以存在这些情况：

1，删除2最好删，把2删除之后，还需要把2的右置成nullpt，不然就是野指针了。

2, 当前节点左为空，父亲指向我的右，当前节点右为空，父亲指向我的左边。

3，叶子结点也可以归类到这种左为空或者右为空，让父亲指向左/右。

3，当左右都不为空的时候不能直接删除，用替换法删除
可以找左子树的最大节点(最右节点)或者右子树的最小节点最左节点)替代它

综上所述：

① 左为空

② 右为空

③ 左右都不为空

1，左为空或右为空

我们不能单单只看cur的左右是否为空，然后直接用parent去指向cur的左右，而是我们需要去观察 cur 属于parent 左边还是右边，如果在左边就用 parent 的左边去指向cur的左或者右，如果在右边就用 parent 的右边去指向cur的左或者右。

右为空和左为空同理。

bool Erase(const K& key)
	{
		Node* parent = nullptr;
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key)
			{
				parent = cur;
				cur = cur->_right;
			}
			else if (key < cur->_key)
			{
				parent = cur;
				cur = cur->_left;
			}
			else
			{
				//找到了
                //1,左边为空
				if (cur->_left == nullptr)
				{
					if (parent->_right == cur)
						parent->_right = cur->_right;
					else
						parent->_left = cur->_right;
					delete cur;
				}
                //2，右边为空
				else if (cur->_right == nullptr) 
				{
					if (parent->_left = cur)
						parent->_left = cur->_left;
					else
						parent->_right = cur->_left;
					delete cur;
				}
				else  //3，左右都不为空
				{
					
				}
				return true;
			}
		}
		return false;
	}

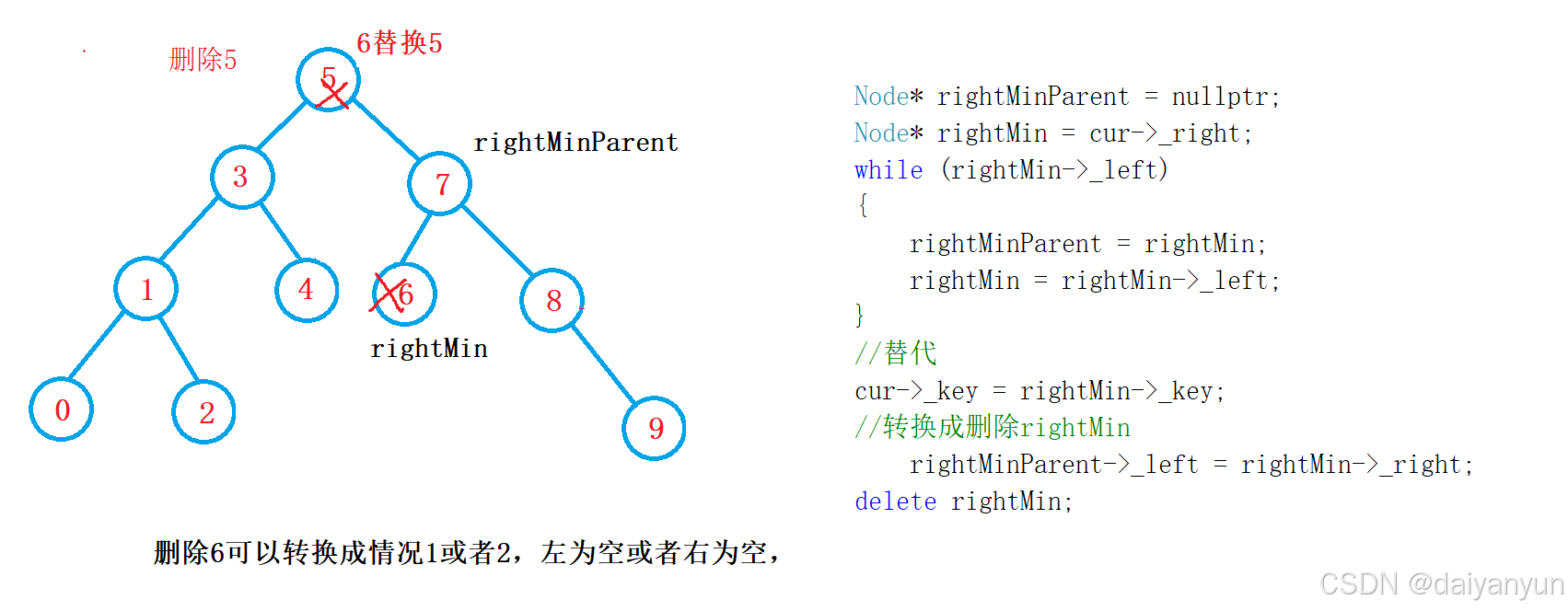
2，左右都不为空

左右都不为空：找左树的最大节点，或者右树的最小节点，也就是左子树的最右节点，或者右子树的最左节点

bool Erase(const K& key)
	{
		Node* parent = nullptr;
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key)
			{
				parent = cur;
				cur = cur->_right;
			}
			else if (key < cur->_key)
			{
				parent = cur;
				cur = cur->_left;
			}
			else
			{
				//找到了
                //1,左边为空
				if (cur->_left == nullptr)
				{
					if (parent->_right == cur)
						parent->_right = cur->_right;
					else
						parent->_left = cur->_right;
					delete cur;
				}
                //2，右边为空
				else if (cur->_right == nullptr) 
				{
					if (parent->_left = cur)
						parent->_left = cur->_left;
					else
						parent->_right = cur->_left;
					delete cur;
				}
				else  //3，左右都不为空
				{
					Node* rightMinParent = nullptr;
                    Node* rightMin = cur->_right;
                    while (rightMin->_left) 
                    {
	                    rightMinParent = rightMin;
	                    rightMin = rightMin->_left;
                    }
                    //替代
                    cur->_key = rightMin->_key;
                    //转换成删除rightMin 
	                rightMinParent->_left = rightMin->_right;
                    delete rightMin;
				}
				return true;
			}
		}
		return false;
	}

假设我一上来就删除 7 这棵树存在问题

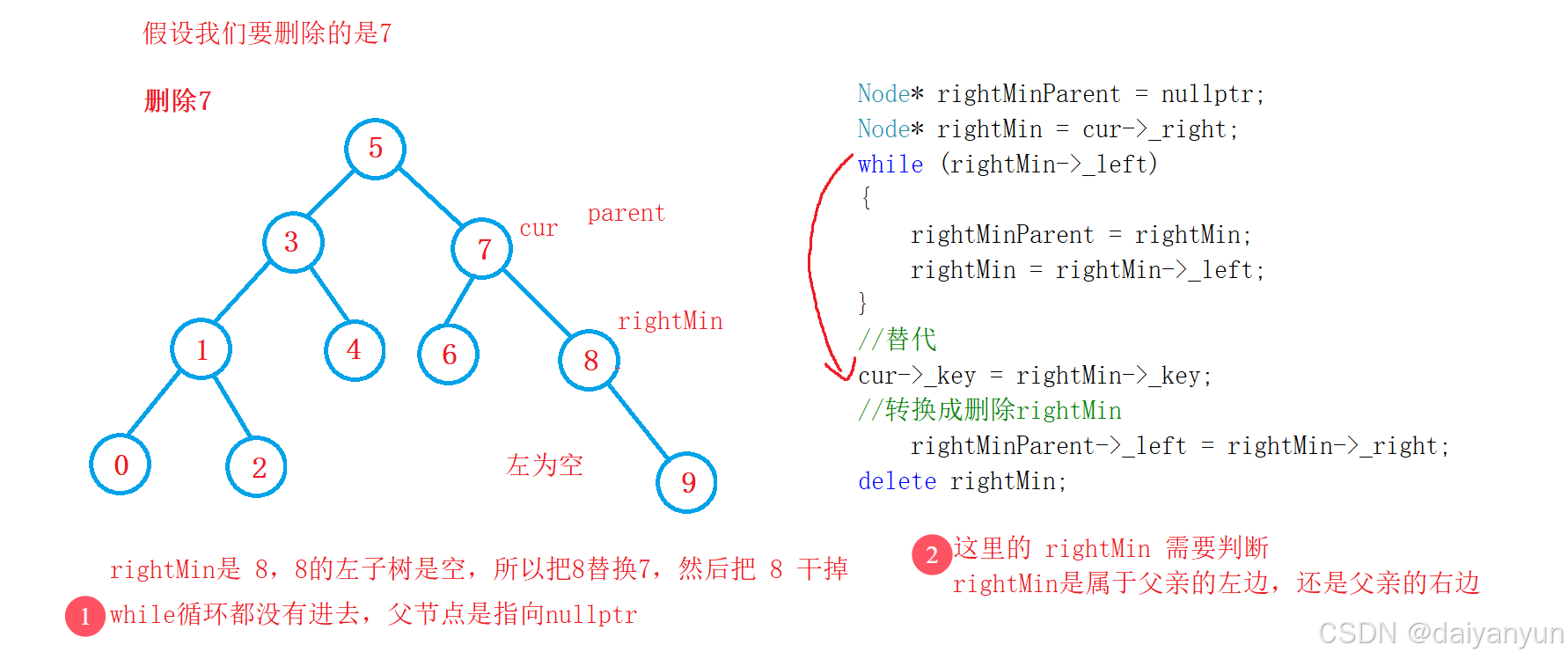
纠正后的代码：

Node* rightMinParent = cur;
Node* rightMin = cur->_right;
while (rightMin->_left) 
{
	rightMinParent = rightMin;
	rightMin = rightMin->_left;
}
//替代
cur->_key = rightMin->_key;
//转换成删除rightMin (rightMin是左为空，父亲指向它的右边)
if (rightMin == rightMinParent->_left) 
	rightMinParent->_left = rightMin->_right;
else
	rightMinParent->_right = rightMin->_right;
delete rightMin;

如果把这棵树删空也会存在问题

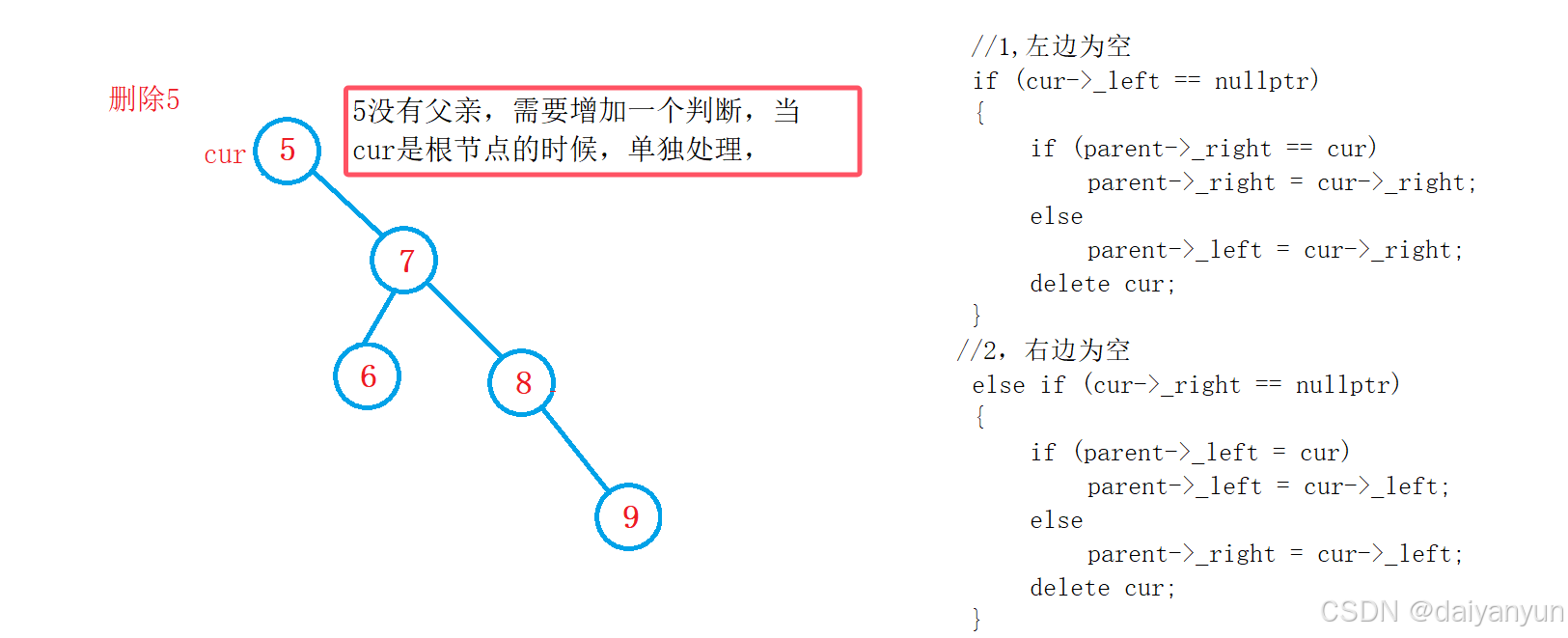
纠正后的代码：

if (cur->_left == nullptr)
{
	if (cur == _root)  //当删除的是根节点的时候
	{
		_root = cur->_right;
	}
	else 
	{
		if (parent->_right == cur)
			parent->_right = cur->_right;
		else
			parent->_left = cur->_right;
	}
	delete cur;
}
else if (cur->_right == nullptr) 
{
	if (cur == _root)   //当删除的是根节点的时候
	{
		_root = cur->_left;  
	}
	else 
	{
		if (parent->_left == cur)
			parent->_left = cur->_left;
		else
			parent->_right = cur->_left;
	}
	delete cur;
}

3，删除的完整代码：

//删除
bool Erase(const K& key) 
{
	Node* parent = nullptr;
	Node* cur = _root;
	while (cur)
	{
		if (key > cur->_key) 
		{
			parent = cur;
			cur = cur->_right;
		}
		else if (key < cur->_key) 
		{
			parent = cur;
			cur = cur->_left;
		}
		else 
		{
			//找到了，开始删除
			// 1、左为空
			// 2、右为空
			// 3、左右都不为空
			if (cur->_left == nullptr) 
			{
				if (cur == _root) 
				{
					_root = cur->_right;
				}
				else 
				{
					if (parent->_right == cur)
						parent->_right = cur->_right;
					else
						parent->_left = cur->_right;
				}
				delete cur;
			}
			else if (cur->_right == nullptr) 
			{
				if (cur == _root)
				{
					_root = cur->_left;
				}
				else 
				{
					if (parent->_left == cur)
						parent->_left = cur->_left;
					else
						parent->_right = cur->_left;
				}
				delete cur;
			}
			else 
			{
				Node* rightMinParent = cur;
				Node* rightMin = cur->_right;
				while (rightMin->_left) 
				{
					rightMinParent = rightMin;
					rightMin = rightMin->_left;
				}
				//替代
				cur->_key = rightMin->_key;
				//转换成删除rightMin (rightMin是左为空，父亲指向它的右边)
				if (rightMin == rightMinParent->_left) 
					rightMinParent->_left = rightMin->_right;
				else 
					rightMinParent->_right = rightMin->_right;
				delete rightMin;
			}
			return true;
		}
	}
	return false;
}

二叉搜索树的完整代码

BSTree.h

#pragma once
template<class K>
struct BSTreeNode
{
	BSTreeNode<K>* _left;
	BSTreeNode<K>* _right;
	K _key;
	BSTreeNode(const K& key) 
		:_left(nullptr)
		,_right(nullptr)
		,_key(key)
	{}
};

template <class K>  //key 关键字，进行比较
class BSTree  //Binary Search Tree
{
	typedef BSTreeNode<K> Node;
public:
	//插入
	bool Insert(const K& key) 
	{
		//当树是空树的时候
		if (_root == nullptr) 
		{
			_root = new Node(key);
			return true;
		}
		//树不为空的时候
		Node* parent = nullptr;
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key) 
			{
				parent = cur;
				cur = cur->_right;	
			}
			else if (key < cur->_key) 
			{
				parent = cur;
				cur = cur->_left;
			}
			else
			{
				return false;
			}
		}
		cur = new Node(key);
		if (key > parent->_key)
			parent->_right = cur;
		else
			parent->_left = cur;
		return true;
	}
	//查找
	bool Find(const K& key) 
	{
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key) 
			{
				cur = cur->_right;
			}
			else if (key < cur->_key) 
			{
				cur = cur->_left;
			}
			else 
			{
				return true;
			}
		}
		return false;
	}
	//删除
	bool Erase(const K& key) 
	{
		Node* parent = nullptr;
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key) 
			{
				parent = cur;
				cur = cur->_right;
			}
			else if (key < cur->_key) 
			{
				parent = cur;
				cur = cur->_left;
			}
			else 
			{
				//找到了，开始删除
				// 1、左为空
				// 2、右为空
				// 3、左右都不为空
				if (cur->_left == nullptr) 
				{
					if (cur == _root) 
					{
						_root = cur->_right;
					}
					else 
					{
						if (parent->_right == cur)
							parent->_right = cur->_right;
						else
							parent->_left = cur->_right;
					}
					delete cur;
				}
				else if (cur->_right == nullptr) 
				{
					if (cur == _root)
					{
						_root = cur->_left;
					}
					else 
					{
						if (parent->_left == cur)
							parent->_left = cur->_left;
						else
							parent->_right = cur->_left;
					}
					delete cur;
				}
				else 
				{
					Node* rightMinParent = cur;
					Node* rightMin = cur->_right;
					while (rightMin->_left) 
					{
						rightMinParent = rightMin;
						rightMin = rightMin->_left;
					}
					//替代
					cur->_key = rightMin->_key;
					//转换成删除rightMin (rightMin是左为空，父亲指向它的右边)
					if (rightMin == rightMinParent->_left) 
						rightMinParent->_left = rightMin->_right;
					else 
						rightMinParent->_right = rightMin->_right;
					delete rightMin;
				}
				return true;
			}
		}
		return false;
	}
	//中序遍历
	void _InOrder(Node* root) 
	{
		if (root == nullptr)
			return;
		_InOrder(root->_left);
		cout << root->_key << " ";
		_InOrder(root->_right);
	}
	void InOrder() 
	{
		_InOrder(_root);
		cout << endl;
	}
private:
	Node* _root = nullptr;
};

void TestBSTree() 
{
	BSTree<int> t;
	int a[] = { 5,3,4,1,7,8,2,6,0,9 };
	for (auto e : a) 
	{
		t.Insert(e);
	}
	t.InOrder();
	//1.上来我就删除7,有问题
	t.Erase(7);
	t.InOrder();
	t.Erase(8);
	t.InOrder();
	//2.把这棵树删空，也会存在问题
	/*for (auto e : a) 
	{
		t.Erase(e);
	}
	t.InOrder();*/
	叶子
	t.Erase(2);
	t.InOrder();
	左为空或者右为空
	t.Erase(8);
	t.Erase(1);
	t.InOrder();
	左右都不为空
	t.Erase(5);
	t.InOrder();
}

test.cpp

#define _CRT_SECURE_NO_WARNINGS 1
#include <iostream>
using namespace std;
#include<string>
#include "BSTree.h"
int main()
{
	TestBSTree();
	return 0;
}

---

二叉搜索树的应用

Key 模型

• 在 Key 模型中，数据主要围绕一个关键标识符（Key）来组织。通常这个 Key 是一个唯一的标识，用于快速检索数据。例如，在一个简单的学生信息系统中，学生的学号可以作为 Key。系统可以根据学号快速查找对应的学生信息，但是可能存储的信息相对比较单一，主要就是和这个 Key 直接相关的内容。
• 它类似于一个索引，重点在于通过这个唯一的标识来定位某个特定的数据项。
• 以上二叉搜索树的实现使用的就是key模型

Key-Value 模型

• Key - Value 模型则是由一个 Key 和一个与之对应的 Value 组成的键值对。Key 仍然用于检索，但是 Value 可以是各种各样的数据结构，如字符串、数字、对象、数组等。比如在一个缓存系统中，Key 可以是一个 URL，Value 则是这个 URL 对应的网页内容。
• 这种模型更强调数据的关联性，Key 和 Value 共同构成了一个完整的数据单元，Value 的内容可以非常丰富，并且 Key 和 Value 之间存在一种明确的对应关系。
• Key 通常是设计为唯一的标识符，用于精确地定位和区分不同的键值对。而 Value 可以是相同的。例如，在一个记录用户购物偏好的系统中，Key 可以是用户的唯一标识（如用户 ID），Value 是用户喜欢的商品类别。多个用户（不同的 Key）可能都喜欢相同的商品类别（相同的 Value）。
• 实际中 Key-Value模型应用广泛。

改造二叉搜索树为KV结构

BSTree.h

#pragma once
// Key-Value 模型
template<class K, class V>
struct BSTreeNode
{
	BSTreeNode<K, V>* _left;
	BSTreeNode<K, V>* _right;
	K _key;
	V _value;
	BSTreeNode(const K& key, const V& value)
		:_left(nullptr)
		, _right(nullptr)
		, _key(key)
		, _value(value)
	{}
};

template <class K, class V>  //key 关键字，进行比较
class BSTree  //Binary Search Tree
{
	typedef BSTreeNode<K, V> Node;
public:
	//插入
	bool Insert(const K& key, const V& value)
	{
		//当树是空树的时候
		if (_root == nullptr)
		{
			_root = new Node(key, value);
			return true;
		}
		//树不为空的时候
		Node* parent = nullptr;
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key)
			{
				parent = cur;
				cur = cur->_right;
			}
			else if (key < cur->_key)
			{
				parent = cur;
				cur = cur->_left;
			}
			else
			{
				return false;
			}
		}
		cur = new Node(key, value);
		if (key > parent->_key)
			parent->_right = cur;
		else
			parent->_left = cur;
		return true;
	}
	//查找
	Node* Find(const K& key)
	{
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key)
			{
				cur = cur->_right;
			}
			else if (key < cur->_key)
			{
				cur = cur->_left;
			}
			else
			{
				return cur;
			}
		}
		return nullptr;
	}
	//删除
	bool Erase(const K& key)
	{
		Node* parent = nullptr;
		Node* cur = _root;
		while (cur)
		{
			if (key > cur->_key)
			{
				parent = cur;
				cur = cur->_right;
			}
			else if (key < cur->_key)
			{
				parent = cur;
				cur = cur->_left;
			}
			else
			{
				//找到了，开始删除
				// 1、左为空
				// 2、右为空
				// 3、左右都不为空
				if (cur->_left == nullptr)
				{
					if (cur == _root)
					{
						_root = cur->_right;
					}
					else
					{
						if (parent->_right == cur)
							parent->_right = cur->_right;
						else
							parent->_left = cur->_right;
					}
					delete cur;
				}
				else if (cur->_right == nullptr)
				{
					if (cur == _root)
					{
						_root = cur->_left;
					}
					else
					{
						if (parent->_left == cur)
							parent->_left = cur->_left;
						else
							parent->_right = cur->_left;
					}
					delete cur;
				}
				else
				{
					Node* rightMinParent = cur;
					Node* rightMin = cur->_right;
					while (rightMin->_left)
					{
						rightMinParent = rightMin;
						rightMin = rightMin->_left;
					}
					//替代
					cur->_key = rightMin->_key;
					//转换成删除rightMin (rightMin是左为空，父亲指向它的右边)
					if (rightMin == rightMinParent->_left)
						rightMinParent->_left = rightMin->_right;
					else
						rightMinParent->_right = rightMin->_right;
					delete rightMin;
				}
				return true;
			}
		}
		return false;
	}
	//中序遍历
	void _InOrder(Node* root)
	{
		if (root == nullptr)
			return;
		_InOrder(root->_left);
		cout << root->_key << ":" << root->_value << endl;
		_InOrder(root->_right);
	}
	void InOrder()
	{
		_InOrder(_root);
		cout << endl;
	}
private:
	Node* _root = nullptr;
};

void TestBSTree()
{
    //输入单词，查找单词对应的中文翻译
	/*BSTree<string, string> dict;
	dict.Insert("sort", "排序");
	dict.Insert("string", "字符串");
	dict.Insert("tree", "树");
	dict.Insert("insert", "插入");
	string str;
	while (cin >> str)
	{
		BSTreeNode<string, string>* ret = dict.Find(str);
		if (ret)
		{
			cout << ret->_value << endl;
		}
		else
		{
			cout << "无此单词" << endl;
		}
	}*/

	//以后很常用,统计水果的个数
	string strArr[] = { "西瓜","西瓜" ,"樱桃","苹果","香蕉","西瓜" ,"西瓜","哈密瓜" ,"西瓜" ,"西瓜" };
	BSTree<string, int> countTree;
	for (auto str : strArr)
	{
		BSTreeNode<string, int>* ret = countTree.Find(str);
		if (ret == nullptr)
		{
			countTree.Insert(str, 1);
		}
		else
		{
			ret->_value++;
		}

	}
	countTree.InOrder();
}

test.cpp

#define _CRT_SECURE_NO_WARNINGS 1
#include <iostream>
using namespace std;
#include<string>
#include "BSTree.h"
int main()
{
	TestBSTree();
	return 0;
}

二叉搜索树的性能分析

最好情况：

• 对于平衡的二叉搜索树，插入操作首先需要找到插入位置。因为树是平衡的，这个查找过程类似于查找操作，时间复杂度为 O(logN)。
• 找到位置后，插入新节点的操作本身时间复杂度为O(1) （只需要修改指针来连接新节点）。所以，整体插入操作在最好情况下的时间复杂度为O(logN)。

最坏情况：

• 当二叉搜索树退化为链表时，插入操作需要先遍历链表找到合适的插入位置。例如，若按照从小到大的顺序插入节点，要插入一个新的最大值，需要遍历到链表的末尾。此时，插入操作的时间复杂度为 O(N)。

问题：如果退化成单支树，二叉搜索树的性能就失去了。那能否进行改进，不论按照什么次序插入关键码，二叉搜索树的性能都能达到最优？那么就有我们后续学习的AVL树和红黑树。