文章目录
算法介绍
快速排序是一种高效的排序算法,由英国计算机科学家C. A. R. Hoare在1960年提出,是对冒泡排序算法的一种改进。
该算法采用 分治策略 ,其基本思想:
选择一个基准元素(pivot),通过基准元素将待排序的序列分成两部分,一部分包含所有小于基准元素的元素,另一部分包含所有大于基准元素的元素,然后递归地对这两部分进行快速排序,最终使整个数组成为一个有序的序列。
算法分析
核心思想
- 选择基准:从数组中选择一个元素作为基准(pivot)。通常可以选择第一个元素、最后一个元素、随机元素或中间元素。
- 分区操作 :
- 遍历数组,将小于基准的元素移动到基准左侧,大于基准的元素移动到右侧。这样基准元素就会被放置到其正确的排序位置。
- 此步骤会让数组分成了两部分:左侧(小于基准)和右侧(大于基准)。
- 递归排序 :
- 对于基准位置左侧和右侧的两个子数组,分别递归地运用快速排序。
- 递归结束的条件是子数组的长度小于或等于 1,此时它们已经有序。
- 合并结果:合并已经排序好的子数组与基准,得到一个完整的有序数组。
三个版本运行过程
以数列 a[] = {40, 20, 30, 60, 10, 50} 作为分析案例;
挖坑法
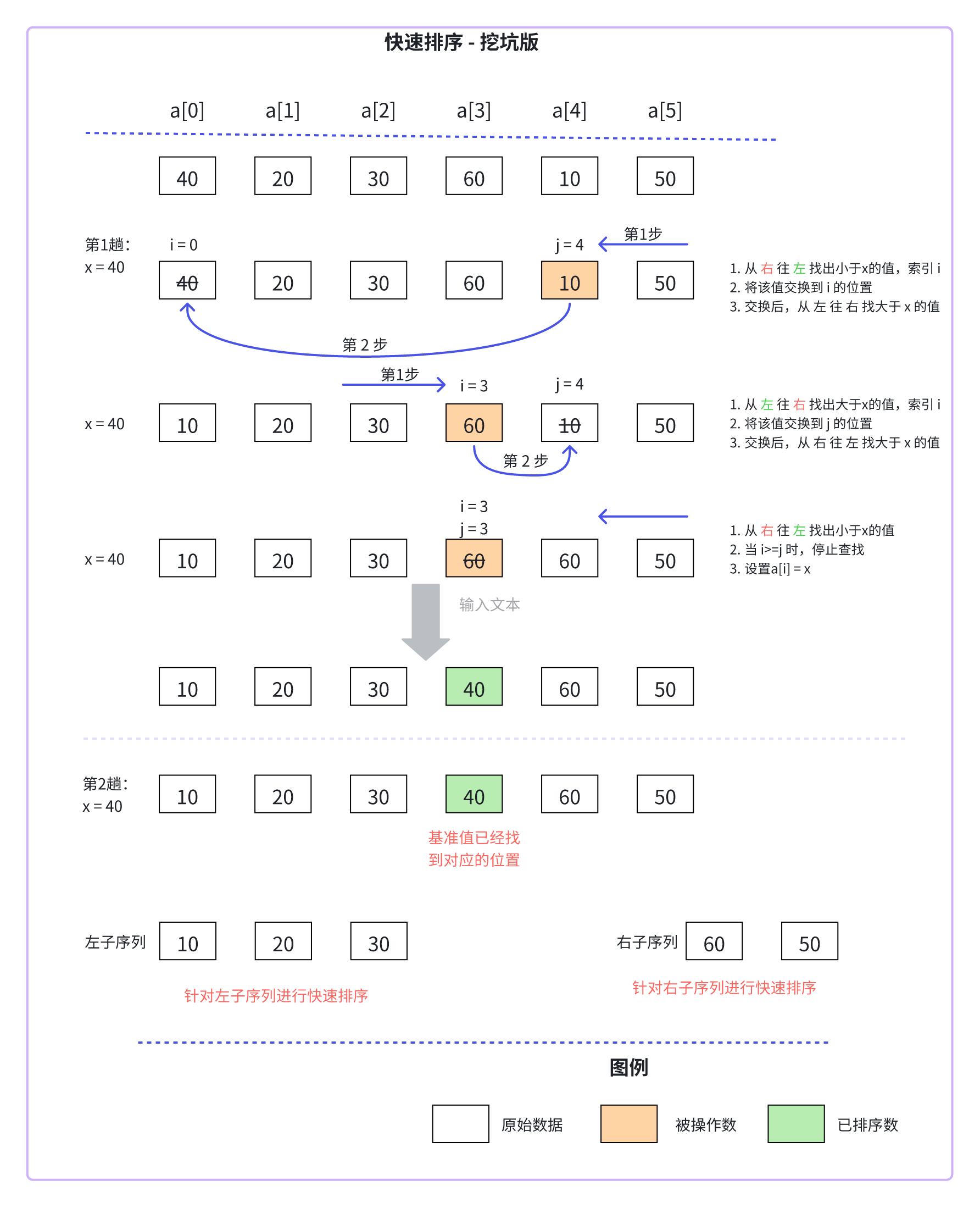
分析:
x 取索引为0 的最左值 为 40,即x = 40。
- 从"右 --> 左"查找小于x的数: 找到满足条件的数a[j]=10,此时 j=4,i=0;然后将a[j]赋值a[i],即将 a[4] 赋值给 a[0];接着从左往右遍历。
- 从"左 --> 右"查找大于x的数: 找到满足条件的数a[i]=60,此时 i=3,j=4;然后将a[i]赋值a[j],即将 a[3] 赋值给 a[4];接着从右往左遍历。
- 从"右 --> 左"查找小于x的数: 没有找到满足条件的数。当i>=j时,停止查找;然后将x赋值给a[i],即将 40 赋值给 a[3]。此趟遍历结束!
代码:
c
#include <stdio.h>
/**
* Quick sort by C Lang.
*
* params:
* arr -- the data array
* left -- the first index of arr
* right -- the last index of arr
*
*/
void quickSort(int arr[], int left, int right)
{
// the arr is empty or left one element
if (left >= right)
{
return;
}
// init data
int pivot = arr[left];
int i = left, j = right;
while (i < j)
{
// right -> left: find the first element less than pivot
// notice: when arr[j] meet the condition `arr[j] < pivot`, it will break the while loop, indicate that current element is less than pivot.
while (i < j && arr[j] > pivot)
j--;
if (i < j)
{
arr[i++] = arr[j];
}
// left -> right: find the first element great than pivot
while (i < j && arr[i] < pivot)
i++;
if (i < j)
{
arr[j--] = arr[i];
}
arr[i] = pivot; // The element has benn correctly placed in its corresponding position
quickSort(arr, left, i - 1); // Recursive invoke in the left sub array
quickSort(arr, i + 1, right); // Recursive invoke in the right sub array
}
}
/**
* show array
*/
void printArray(int array[], int n)
{
for (size_t i = 0; i < n; i++)
{
printf("%d ", array[i]);
}
printf("\n\n");
}
int main()
{
int arr[] = {40, 20, 30, 60, 10, 50};
// calculate the length of a array
int n = sizeof(arr) / sizeof(arr[0]);
printf("before sort: \n");
printArray(arr, n);
// The third param must be `n -1`
quickSort(arr, 0, n - 1);
printf("after sort: \n");
printArray(arr, n);
return 0;
}代码详见:GitHub 快速排序法 挖坑版
Hoare 原版
分析:
- 选择基准值:快速排序的第一步是选择一个基准值(pivot)。通常可以选择数组的第一个元素,但为了提高排序效率,有时也会选择随机元素或使用其他策略。
- 划分过程:使用两个指针,左指针和右指针,分别从数组的两端开始向中间移动。
- 具体步骤:
- 左指针移动:左指针从左向右移动,直到找到一个大于或等于基准值的元素。
- 右指针移动:右指针从右向左移动,直到找到一个小于或等于基准值的元素。
- 交换元素:如果左指针指向的元素大于基准值,且右指针指向的元素小于基准值,则交换这两个元素。
- 继续移动指针:继续移动左右指针,直到它们相遇。
- 交换基准值:最后,将基准值与右指针指向的元素交换,确保基准值左侧的所有元素都不大于基准值,右侧的所有元素都不小于基准值。
代码:
c
#include <stdio.h>
/**
* swap two number
*/
void swap(int *a, int *b)
{
int temp = *a;
*a = *b;
*b = temp;
}
/**
* Quick sort by C Lang.
*
* params:
* arr -- the data array
* left -- the first index of arr
* right -- the last index of arr
*
*/
int partition(int arr[], int left, int right)
{
// init data
int pivotIndex = left;
int i = left, j = right;
while (i < j)
{
// right -> left: find the first element less than pivot
while (i < j && arr[j] > arr[pivotIndex])
{
j--;
}
// left -> right: find the first element great than pivot
while (i < j && arr[i] < arr[pivotIndex])
{
i++;
}
// after upper two loop, indicate that it is able to swap
if (i < j)
swap(&arr[i], &arr[j]);
}
// Swap the pivot with the element pointed by the right pointer
swap(&arr[pivotIndex], &arr[j]);
return j;
}
void quickSort(int arr[], int left, int right)
{
if (left < right)
{
int pivotIndex = partition(arr, left, right);
quickSort(arr, left, pivotIndex - 1);
quickSort(arr, pivotIndex + 1, right);
}
}
/**
* show array
*/
void printArray(int array[], int n)
{
for (size_t i = 0; i < n; i++)
{
printf("%d ", array[i]);
}
printf("\n\n");
}
int main()
{
int arr[] = {40, 20, 30, 60, 10, 50};
// calculate the length of a array
int n = sizeof(arr) / sizeof(arr[0]);
printf("before sort: \n");
printArray(arr, n);
// The third param must be `n -1`
quickSort(arr, 0, n - 1);
printf("after sort: \n");
printArray(arr, n);
return 0;
}代码详见:GitHub 快速排序法 hoare版
前后指针法
前后指针法(也称为荷兰国旗问题解法)是快速排序中的一种高效实现方式。在这个方法中,通常将数组划分为三个部分:
- 小于基准值的元素
- 等于基准值的元素
- 大于基准值的元素。
分析:
-
变量初始化:
- key 是基准元素的索引,初始为 left。
- prev 是逻辑分区点之前的最后一个小于基准的元素的索引,开始等于 left。
- cur 是当前遍历的元素索引,从 left + 1 开始。
-
分区逻辑:
- 使用 while 循环遍历数组,从 cur = left + 1 到 right。
- 如果 a[cur] < a[key],则 prev 增加(指向下一个元素),并交换 a[prev] 和 a[cur],确保小于基准的元素移动到前面。
- 即使 prev 和 cur 相等,也进行交换,这一步是为了简化逻辑,即不需要特判 prev 和 cur 相等的情况。实际上在交换时什么也不会变。
-
基准交换:
循环结束后,将基准值与 a[prev] 交换,把基准放置在正确的位置。
代码
c
#include <stdio.h>
/**
* swap two number
*/
void swap(int* a, int* b) {
int temp = *a;
*a = *b;
*b = temp;
}
/**
* Quick sort by C Lang.
*
* params:
* arr -- the data array
* left -- the first index of arr
* right -- the last index of arr
*
*/
int partition(int arr[], int left, int right) {
int pivotIndex = left;
int prev = left;
int cur = left + 1;
while (cur <= right) {
if (arr[cur] < arr[pivotIndex] && ++prev != cur) {
swap(&arr[prev], &arr[cur]);
}
cur++;
}
swap(&arr[prev], &arr[pivotIndex]);
return prev;
}
void quickSort(int arr[], int left, int right) {
if (left < right) {
int pivot_index = partition(arr, left, right);
quickSort(arr, left, pivot_index - 1);
quickSort(arr, pivot_index + 1, right);
}
}
void print_array(int arr[], int size) {
for (int i = 0; i < size; i++) {
printf("%d ", arr[i]);
}
printf("\n");
}
int main() {
int arr[] = {30, 40, 60, 10, 20, 50};
int n = sizeof(arr) / sizeof(arr[0]);
printf("Before sorting:\n");
print_array(arr, n);
quickSort(arr, 0, n - 1);
printf("After sorting:\n");
print_array(arr, n);
return 0;
}代码详见:GitHub 快速排序法 前后指针版
算法稳定性和复杂度
稳定性
这一点是因为在分区过程中,元素的相对顺序可能会改变。
具体来说,当我们交换元素以确保小于基准的元素在前,大于基准的在后时,相同元素的相对位置可能改变。
例如,如果两个相等的元素分别在基准的两侧,交换后它们的位置关系就被打破了。
举个例子:
示例数组:[ 3 a 3_a 3a, 1, 2, 3 b 3_b 3b]
这里的 3 a 3_a 3a, 3 b 3_b 3b 是两个不同位置的相同元素(相同的值但用下标区分,用于说明其在数组中的初始位置)。
根据实现可能不交换,但假设实现中等于基准的情况下它也移动,这里交换 3 b 3_b 3b, 3 a 3_a 3a得到数组 [ 3 b 3_b 3b, 1, 2, 3 a 3_a 3a],顺序已经发生了变更。
时间复杂度
平均情况O(nlogn)
基本操作
快速排序的基本操作是划分和递归调用。
操作次数
- 划分操作:每次划分操作将数组分为两部分。在平均情况下,我们假设每次划分都能将数组大致分为两个相等的部分。这意味着每次划分操作都会遍历整个数组一次,操作次数为 n。
- 递归操作:由于每次划分都将数组分为两个大致相等的部分,所以每次递归调用处理的数组大小大约是前一次的一半。这个过程会一直持续到子数组的大小为1,此时不需要再进行划分。
对于大小为 n 的数组,快速排序的递归关系可以表示为:
T ( n ) = 2 T ( n 2 ) + n T(n) = 2T\left(\frac{n}{2}\right) + n T(n)=2T(2n)+n
- T(n)是对大小为 n 的数组进行排序所需的总操作次数。
- T( n 2 \frac{n}{2} 2n)表示对两个大小为 n 2 \frac{n}{2} 2n的子数组进行排序所需的操作次数。
- n 是当前划分操作所需的总比较次数。
大O表示法
这种形式可以用主定理求解,符合主定理的标准形式,所以复杂度为:O(n log n)
最差情况O( n 2 n^2 n2)
基本操作
快速排序的基本操作是划分和递归调用。
操作次数
在最坏情况下,每次划分操作只能将数组分成一个大小为 n−1 的部分和一个大小为 0 的部分(例如,当数组已经有序或每次都选择最大或最小元素作为基准时)。在这种情况下,递归关系式变为:
T ( n ) = T ( n − 1 ) + n T(n) = T(n - 1) + n T(n)=T(n−1)+n
- T(n - 1)是对大小为 n−1 的子数组进行排序所需的操作次数。
- n 是当前划分操作所需的总比较次数。
上述等价于
T ( n ) = n + ( n − 1 ) + ( n − 2 ) + ( n − 3 ) + . . . + 2 + 1 = ( n + 1 ) n 2 = n 2 2 + n 2 T(n)= n + (n-1) + (n-2) + (n-3) + ... + 2 + 1 = \frac{(n + 1)n}{2} = \frac{n^2}{2} + \frac{n}{2} T(n)=n+(n−1)+(n−2)+(n−3)+...+2+1=2(n+1)n=2n2+2n
大O表示法
上述用大O表示法为:O( n 2 n^2 n2)
空间复杂度
快速排序是一种递归算法。每次递归调用时,程序会在栈上保存当前调用的状态信息:
- 平均情况下:
- 快速排序以较好的概率实现了平均分区,递归调用的深度大约是树的高度,即 O(logn)。
- 每次递归在栈上保存的信息量是常数级别的,例如数组的起始和结束索引、基准元素信息等。
- 最坏情况下:
- 如果每次选择的基准是最小或最大元素,导致极端不平衡的分区,递归深度达到 O(n)。
- 这种情况会在已经排序的数组或逆序数组上发生。