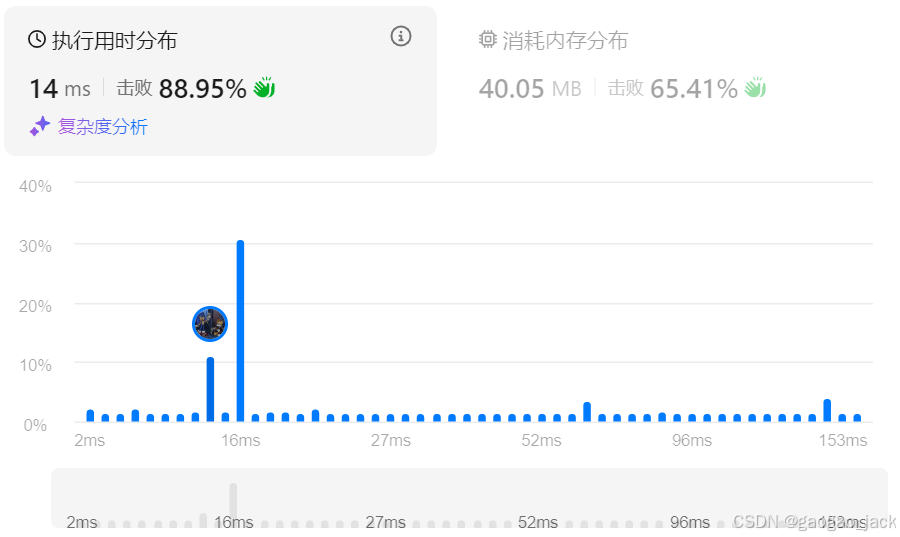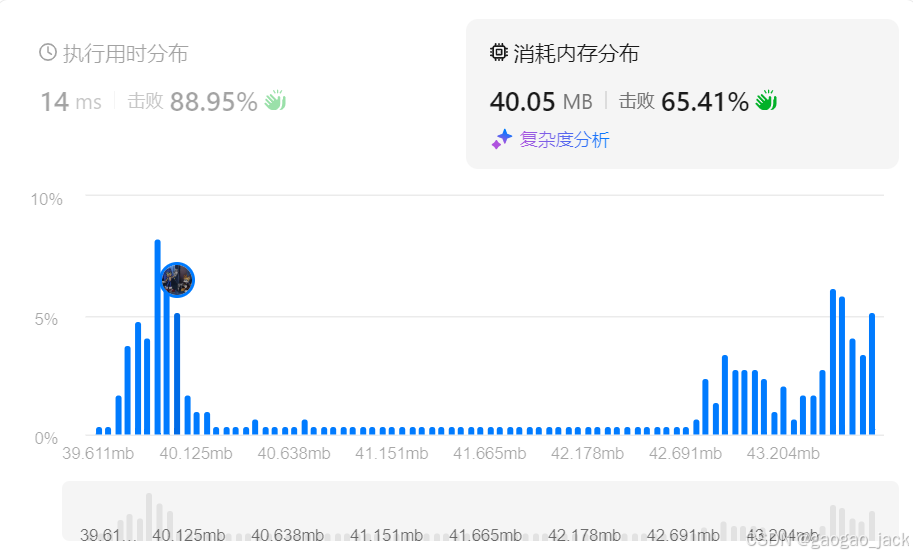
代码:
方法一:平凡解法(最直观但时间复杂度最高)
java
class Solution {
public int nonSpecialCount(int l, int r) {
int res=r-l+1;//初始不是特殊数字的答案为[l,r]范围内数字总数
for(int i=(int)Math.ceil(Math.sqrt(l));i<=(int)Math.floor(Math.sqrt(r));i++){
if(isPrime(i)) res--;//如果是质数,说明是特殊数字,则不是特殊数字的数-1
}
return res;
}
private boolean isPrime(int number){//判断数字是否是质数
if(number==1) return false;
if(number==2) return true;
for(int i=2;i<=(int)Math.sqrt(number);i++){
if(number%i==0) return false;
}
return true;
}
}**时间复杂度:**对于 nonSpecialCount 方法中的每个i,isPrime 方法被调用一次,其时间复杂度为,由于 i 的范围是
到
,需要计算这些调用的总时间复杂度。
在最坏情况下,当 l=1 且 r 非常大时,nonSpecialCount 方法的时间复杂度主要由以下部分组成:
①循环次数:最多次;②每次循环中调用
isPrime 的时间复杂度为,其中i最大值为
。因此,总时间复杂度可以近似为:
。由于
是递增的,并且主要关心的是当 r 非常大时的行为,这个求和可以近似为:
,其中 r 是给定的区间上界。(注:该计算为较宽松的估计,实际上由于 i 的增长速度较慢,并且并不是对每一个 i 都进行到
的检查(而是到
),实际运行时间可能会比该估计要快。但从理论上看,该估计给出了一个上界。)
**空间复杂度:**O(1)

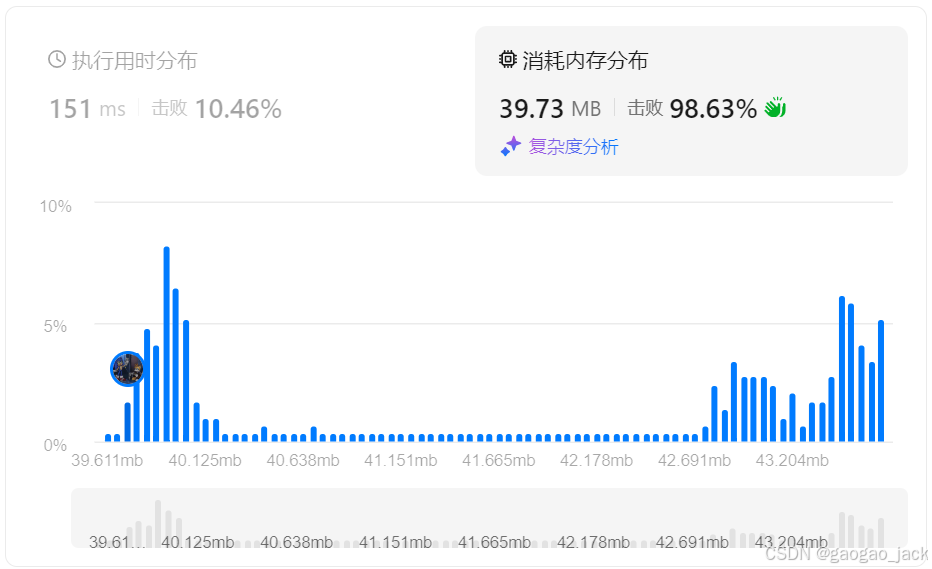
方法二:埃拉托斯特尼筛法(埃氏筛)
原理:"埃拉托斯特尼筛法,简称埃氏筛,是由希腊数学家埃拉托斯特尼提出的筛选质数的算法。埃氏筛的原理是:对于质数 p,所有大于 p 的 p 的倍数都是合数,根据该性质移除所有的合数。
使用埃氏筛得到不超过 upperBound 的所有质数的做法是:使用列表记录从 2 到 upperBound 的所有整数,每次保留列表中的最小整数 p,将 p 保留,并将列表中的所有大于 p 的 p 的倍数都删除,当没有更多的整数可以删除时,剩下的数就是不超过 upperBound 的所有质数。"
java
class Solution {
public int nonSpecialCount(int l, int r) {
int lowerBound = (int) Math.ceil(Math.sqrt(l));//下界
int upperBound = (int) Math.floor(Math.sqrt(r));//上界
boolean[] isPrime = new boolean[upperBound + 1];
Arrays.fill(isPrime, true);
isPrime[0] = false;
isPrime[1] = false;
for(int i = 2; i * i <= upperBound; i++) {
if(isPrime[i]) {
for(int j = i * i; j <= upperBound; j += i) isPrime[j] = false;
}
}
int res = 0;
for(int i = lowerBound; i <= upperBound; i++) {
if(isPrime[i]) res++;
}
return r-l+1-res;//总数减去是特殊数字的数量即为不是特殊数字的数量
}
}时间复杂度:,其中 r 是给定的区间上界。
空间复杂度:,其中 r 是给定的区间上界。
方法三:欧拉筛( 线性筛)->避免重复标记
**参考链接:**https://leetcode.cn/problems/find-the-count-of-numbers-which-are-not-special/solutions/2860320/3233-tong-ji-bu-shi-te-shu-shu-zi-de-shu-enhg/
该方法可看作是方法二的改进版,因为埃氏筛存在重复标记的情况,如果一个合数有多个不同的质因数,则会被标记多次,比如30会被2、3、5分别标记一次。
原理(引用自参考链接):

java
class Solution {
public int nonSpecialCount(int l, int r) {
int lb = (int) Math.ceil(Math.sqrt(l));//下界
int ub = (int) Math.floor(Math.sqrt(r));//上界
List<Integer> primes = new ArrayList<Integer>();
boolean[] isPrime = new boolean[ub+1];
Arrays.fill(isPrime, true);
isPrime[0] = false;
isPrime[1] = false;
for(int i=2;i<=ub;i++) {
if(isPrime[i]) primes.add(i);
for(int prime:primes) {
if(i*prime<=ub) {
isPrime[i*prime]=false;
if(i%prime==0) break;
}
else break;
}
}
int res=0;
for(int i=lb;i<=ub;i++){
if(isPrime[i]) res++;//是特殊数字的数字数量
}
return r-l+1-res;//总数-是特殊数字的数字数量即为题目答案
}
}时间复杂度:,其中 r 是给定的区间上界。
空间复杂度:,其中 r 是给定的区间上界。
方法四:求范围内质数
该方法可看作是方法一的优化。
思路:首先根据题意,特殊数字是指除1和本身外只包含一个质数的数字。即相当于质数的平方。然后对数据进行预处理,判断范围内的质数。对于质数范围,假设右边界r是特殊数字,则必然存在一个质数Math.sqrt(r),即为质数范围最大值。然后完成问题转换,将[l,r]区间单个进行枚举判断,转为根据范围内质数算出特殊数字,并判断是否落在区间内。最后用总数减去特殊数字个数即为题目结果。
java
class Solution {
public int nonSpecialCount(int l, int r) {
int n = (int) Math.sqrt(r) + 1;
boolean[] pir = getPrimeInRange(n);
// 统计特殊数字个数
int res=0;
for (int i = 0; i < pir.length ;i++) {
if (!pir[i]) continue;
// 当前i为质数,num为特殊数字
int num=i*i;
// 判断特殊数字是否落在lr区间内
if (num > r) break;
else if (num < l) continue;
else res++;
}
return r-l+1-res;
}
private static boolean[] getPrimeInRange(int n) {// 函数作用:判断范围内的质数
boolean[] isPrime = new boolean[n];// 使用数组存储该位是否为质数
Arrays.fill(isPrime, true);
isPrime[1] = false;
for(int i=2;i*i<n;i++) {
if(isPrime[i]) {
for (int j = i * i; j < n; j += i) isPrime[j] = false; // 原数是质数,在范围n内累乘迭代,结果均为非质数
}
}
return isPrime;
}
}时间复杂度:
空间复杂度:

方法五:预处理质数(灵神解法)
(该方法较为巧妙)

java
class Solution {
private static final int Max = 31622;
private static final int[] p = new int[Max + 1];
static{
for (int i = 2; i <= Max; i++) {
if (p[i] == 0){// i 是质数
p[i] = p[i-1] + 1;
for (int j = i * i; j <=Max; j += i) p[j] = -1; // 标记 i 的倍数为合数 // 注:如果 MX 比较大,小心 i*i 溢出
} else p[i] = p[i - 1];
}
}
public int nonSpecialCount(int l, int r) {
return r-l+1-(p[(int)Math.sqrt(r)]-p[(int)Math.sqrt(l-1)]);
}
}时间复杂度:,预处理不计。
空间复杂度:,预处理不计。