数据结构(C语言版)-4.树与二叉树

基本概念

• 满二叉树

  • 

• 完全二叉树

• 

二叉树的性质

二叉树的存储结构

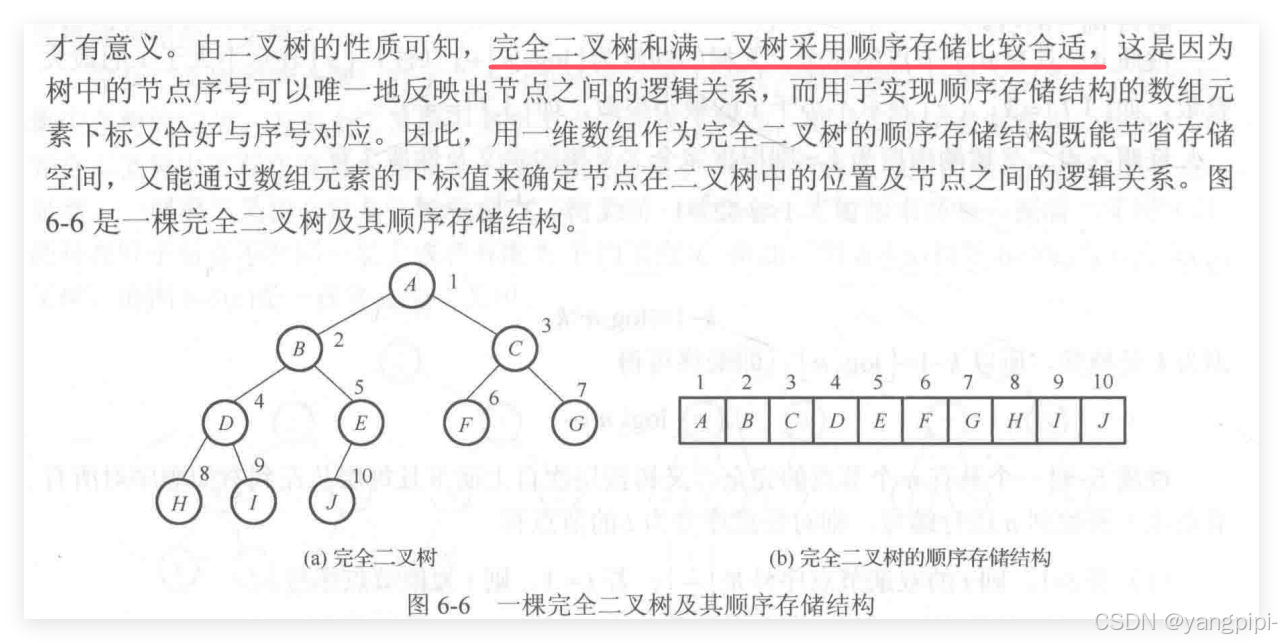
顺序存储结构

链式存储结构

二叉树的遍历(以二叉链表为例)

前序遍历

递归遍历

void Preorder(BSTree* p)
{
	if (p != NULL)
	{
		printf("%c ",p->data);
		Preorder(p->lchild);
		Preorder(p->rchild);
	}
}

非递归遍历

void PreorderNoRecursive(BSTree* p)
{
	/*
		思路: 不断遍历左子树然后将其入栈，知道遇到NULL，弹栈，然后指向弹出元素的右子树
	*/
	std::stack<BSTree*> sta;
	while (p!=NULL || !sta.empty())
	{
		if (p!=NULL)
		{
			printf("%c ", p->data);
			sta.push(p);
			p = p->lchild;
		}
		else
		{
			p = sta.top();
			sta.pop();
			p = p->rchild;
		}
	}
}

中序遍历

递归遍历

void Inorder(BSTree* p)
{
	if (p != NULL)
	{
		Inorder(p->lchild);
		printf("%c ", p->data);
		Inorder(p->rchild);
	}
}

非递归遍历

void InorderNoRecursive(BSTree* p)
{
	/*
		思路: 不断将左子树入栈，直到遇到NULL，弹栈，访问，然后指向弹出元素的右子树
	*/
	std::stack<BSTree*> sta;
	while (p != NULL || !sta.empty())
	{
		if (p != NULL)
		{
			sta.push(p);
			p = p->lchild;
		}
		else
		{
			p = sta.top();
			sta.pop();
			printf("%c ", p->data);
			p = p->rchild;
		}
	}
}

后序遍历

递归遍历

void Postorder(BSTree* p)
{
	if (p != NULL)
	{
		Postorder(p->lchild);
		Postorder(p->rchild);
		printf("%c ", p->data);
	}
}

非递归遍历(困难)

设置访问标志b(一层)

void PostorderNoRecursive1(BSTree* p)
{
	BSTree*  sta[MAXSIZEBS];
	int i = 0, b[MAXSIZEBS];
	sta[0] = NULL;
	do
	{
		if (p != NULL)
		{
			sta[++i] = p;
			b[i] = 0;  // 置*p的右子树未访问过的标志
			p = p->lchild;
		}
		else
		{
			p = sta[i--];
			if (b[i + 1] == 0)
			{
				sta[++i] = p;
				b[i] = 1; // 置*p的右子树已访问的标志
				p = p->rchild;
			}
			else
			{
				printf("%c ",p->data);
				p = NULL;  // 指向*p的指针置空
			}
		}
	} while (p != NULL || i > 0);
}

二层循环

前序-> 后序

先依次将右子树依此压栈，直到右子树为NULL时，再压栈，访问此元素的左子树。最后将得到的元素反向输出即可。

void PostorderNoRecursive(BSTree* p)
{
	std::stack<BSTree*> sta;
	std::vector<char> vec;
	while (p != NULL || !sta.empty())
	{
		if (p != NULL)
		{
			//printf("%c ", p->data);
			vec.push_back(p->data);
			sta.push(p);
			p = p->rchild;
		}
		else
		{
			p = sta.top();
			sta.pop();
			p = p->lchild;
		}
	}

	for (std::vector<char>::const_reverse_iterator it = vec.rbegin(); it != vec.rend(); it++)
	{
		printf("%c ",*it);
	}
}

层次遍历

void Transleve(BSTree* t)
{
	/*
		使用队列，依次访问每一个元素。
	*/

	// 层次遍历的方式一
	//std::queue<BSTree*> de;
	//BSTree* p;
	//if (t != NULL)
	//{
	//	printf("%c ", t->data);
	//	de.push(t);
	//}
	//else 
	//	return;
	//while (!de.empty())
	//{
	//	p = de.front();
	//	de.pop();
	//	if (p->lchild != NULL)
	//	{
	//		printf("%c ", p->lchild->data);
	//		de.push(p->lchild);
	//	}
	//	if (p->rchild != NULL)  //注意这里不是else if
	//	{
	//		printf("%c ", p->rchild->data);
	//		de.push(p->rchild);
	//	}
	//}

	// 层次遍历的方式2：
	std::queue<BSTree*> de;
	BSTree* p;
	if (t != NULL)
		de.push(t);
	else 
		return;
	while (!de.empty())
	{
		p = de.front();
		printf("%c ", p->data);
		de.pop();
		if (p->lchild != NULL)
			de.push(p->lchild);
		if (p->rchild != NULL)
			de.push(p->rchild);
	}

}

练习题

根据条件交换二叉树的左右子树

void Change(BSTree* p)
{
	if (p != NULL)
	{
		if (p->lchild!=NULL&& p->rchild!=NULL&&p->lchild->data > p->rchild->data) // 如果左子树大于右子树的话，就交换左右子树
		{
			BSTree* tmp = p->lchild;
			p->lchild = p->rchild;
			p->rchild = tmp;
		}
		Change(p->lchild);
		Change(p->rchild);
	}
}

查找元素

根据中序非递归查找

// 查找
// 搜素 根据中序非递归遍历查找元素
BSTree* Search_In_NoRecursive(BSTree* p, datatypeBS x)
{
	std::stack<BSTree*> sta;
	while (p != NULL || !sta.empty())
	{
		if (p != NULL)
		{
			sta.push(p);
			p = p->lchild;
		}
		else
		{
			p = sta.top();
			sta.pop();
			//printf("%c ", p->data);
			if (p->data == x)
				return p;
			p = p->rchild;
		}
	}

	return NULL;
}

根据先序递归查找（易错）

BSTree* Search_Pre_Recursive(BSTree* bt, datatypeBS x)
{
	BSTree* p = NULL;
	if (bt != NULL)
	{
		/*printf("%c ", p->data);*/
		if (bt->data == x)
			return bt;
		if (bt->lchild != NULL)
		{
			p = Search_Pre_Recursive(bt->lchild, x);
			if (p != NULL)
				return p;
		}
		if (bt->rchild != NULL)
		{
			p = Search_Pre_Recursive(bt->rchild, x);
			if (p != NULL)
				return p;
		}
			
	}
	return NULL;
}

统计二叉树中叶子节点的个数

递归方法

// 统计叶子节点的个数
int Countleaf(BSTree* p)
{
	if (p == NULL)  // 空二叉树
		return 0;
	if (p->lchild == NULL && p->rchild == NULL) // 叶子节点
		return 1;
	return Countleaf(p->lchild) + Countleaf(p->rchild);
}

非递归方法

int Countleaf_PreorderNoRecursive(BSTree* p)
{
	/*
		思路: 不断遍历左子树然后将其入栈，知道遇到NULL，弹栈，然后指向弹出元素的右子树
	*/
	int count = 0;
	std::stack<BSTree*> sta;
	while (p != NULL || !sta.empty())
	{
		if (p != NULL)
		{
			//printf("%c ", p->data);
			if (p->lchild == NULL && p->rchild == NULL)
				count++;
			sta.push(p);
			p = p->lchild;
		}
		else
		{
			p = sta.top();
			sta.pop();
			p = p->rchild;
		}
	}
	
	return count;
}

求二叉树深度

// 求二叉树的深度
int Depth(BSTree* p)
{
	int lchild = 0, rchild = 0;
	if (p == NULL)
		return 0;
	else {
		lchild = Depth(p->lchild)+1;
		rchild = Depth(p->rchild)+1;
		return lchild > rchild ? lchild : rchild;
	}
}

判断二叉树是否是完全二叉树

• 利用层序遍历，在将一个节点压入队列之前，如果其有右孩子而没有左孩子时则不是满二叉树。

// 判断一个二叉树是否是完全二叉树
int is_complete_binary_tree(BSTree* t)
{
	std::queue<BSTree*> de;
	BSTree* p;
	if (t != NULL)
		de.push(t);
	else
		return 1;
	while (!de.empty())
	{
		p = de.front();
		//printf("%c ", p->data);
		de.pop();
		if (p->lchild != NULL)
			de.push(p->lchild);
		else
		{
			if (p->rchild != NULL)
				return 0;   // 不是完全二叉树
		}
		if (p->rchild != NULL)
			de.push(p->rchild);
	}

	return 1;  // 是完全二叉树
}

找出二叉树中最深的通道

// 求二叉树的最长深度并输出数值
int len = 0;
int longest_len = 0;
void longest_path(BSTree* T, char* path, char* longestpath)
{
	int j, i;
	if (T)//树不空
	{
		if (!T->lchild && !T->rchild)//当遇到叶子结点时，该条路径完毕 
		{
			path[len] = T->data;//把结点数据放入数组 
			if (len > longest_len)//如果长于longest_len就替换 
			{
				for (j = 0; j <= len; j++)//把路径复制到longestpath里面 
					longestpath[j] = path[j];
				longest_len = len;//longest_len更新 
			}
		}
		else//当遇到的不是叶子结点时，该条路径继续 
		{
			path[len++] = T->data;//结点放入数组，len++ 
			longest_path(T->lchild, path, longestpath);
			longest_path(T->rchild, path, longestpath);
			len--;//每次退出递归len-- 
		}
	}
}

注意，定义全局遍历的不要再头文件中定义。应该在一个.c文件中定义并且初始化。如果其他.c文件想使用的话，需要在头文件中使用·extern声明注意注意只是声明不要初始化。

在a.c中 int num = 1; 在a.h中 extern int num; 在b.c中只需要#include"a.h"即可在b.c中使用定义的全局变量num

二叉树的创建

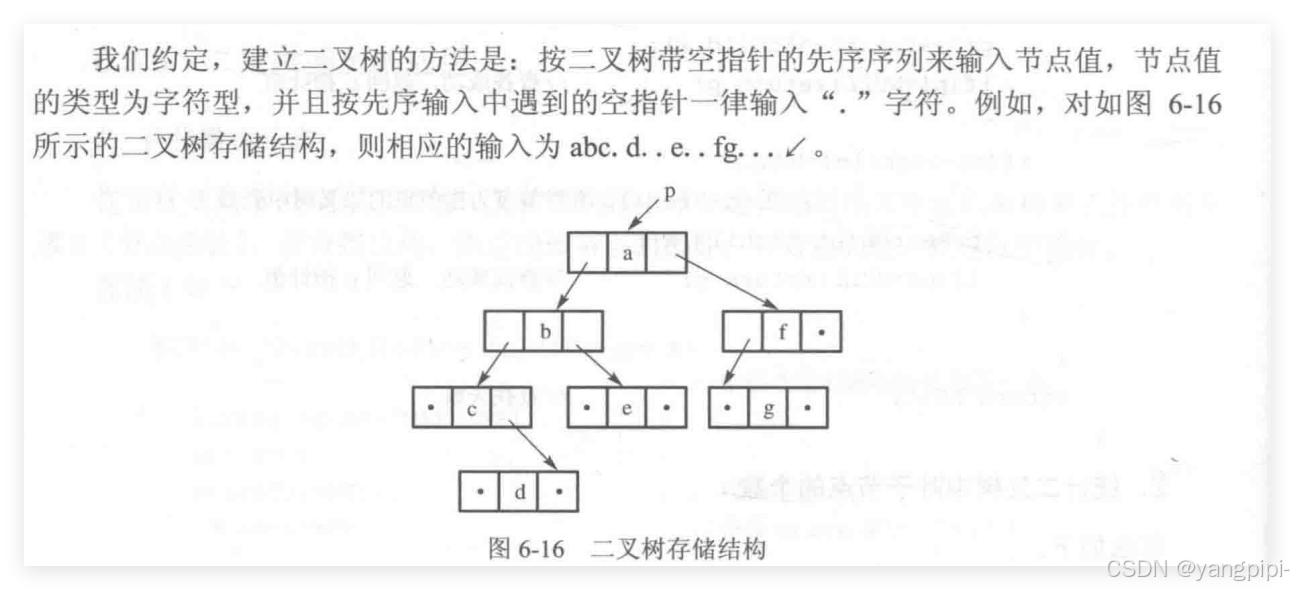
根据先序序列创建

void CreatebByPreorder(BSTree** p)
{
	char ch;
	scanf("%c",&ch);
	if (ch != '.')
	{
		*p = (BSTree*)malloc(sizeof(BSTree));
		(*p)->data = ch;
		CreatebByPreorder(&((*p)->lchild));
		CreatebByPreorder(&((*p)->rchild));
	}
	else
		*p = NULL;
}

由遍历序列恢复二叉树

• 先序+中序 -》 可恢复

• 

• 后序+中序-》可恢复

• 

先序+中序 -》二叉树

在这里插入图片描述

// 根据 先序+中序-》 二叉树 
void Pre_In_Oreder(char pred[], char ind[], int i, int j, int k, int h, BSTree** p)
{
	*p = (BSTree*)malloc(sizeof(BSTree));
	(*p)->data = pred[i]; // 首先构造头结点
	// 从中序序列中找到头结点的位置
	int m = k;
	while (ind[m] != pred[i])
		m++;
	// 构造左子树
	if (m == k) // 左子树为NULL
		(*p)->lchild = NULL;
	else
		Pre_In_Oreder(pred,ind,i+1, i+m-k,k,m-1,&(*p)->lchild);

	// 构造右子树
	if (m == h) // 右子树为NULL
		(*p)->rchild = NULL;
	else
		Pre_In_Oreder(pred, ind, i + m - k+1, j , m+1, h, &(*p)->rchild);
}

• 主要是每次遍历先序序列的范围
  

int main()
{
	BSTree* tree1;
	char pred[] = "acbgdehfji";
	char ind[] = "cgbahedjfi";
	Pre_In_Oreder(pred, ind, 0, 9, 0, 9, &tree1); //void Pre_In_Oreder(char pred[], char ind[], int i, int j, int k, int h, BSTree** p)
	
	printf("\nTransleve遍历结果:");
	Transleve(tree1);
	printf("\nPostorder遍历结果:");
	Postorder(tree1);
	return 0;
}

后序+中序 -》二叉树

void Post_In_Oreder(char post[], char ind[], int i, int j, int k, int h, BSTree** p)
{
	*p = (BSTree*)malloc(sizeof(BSTree));
	(*p)->data = post[j]; // 首先构造头结点
	// 从后序序列中找到头结点的位置
	int m = k;
	while (ind[m] != post[j])
		m++;
	
	// 构造左子树
	if (m == k) // 左子树为NULL
		(*p)->lchild = NULL;
	else
		Post_In_Oreder(post, ind, i, i + m - k-1, k, m - 1, &(*p)->lchild);

	// 构造右子树
	if (m == h) // 右子树为NULL
		(*p)->rchild = NULL;
	else
		Post_In_Oreder(post, ind, i + m - k, j-1, m + 1, h, &(*p)->rchild);
}

线索二叉树

线索化二叉树

// 线索二叉树------------------------------------------------
// 中序线索二叉树
void Thread(TBTree* p)  // 对二叉树的进行线索化
{
	if (p != NULL)
	{
		Thread(p->lchild);
		if (p->lchild == NULL)
		{
			p->ltag = 1;
			p->lchild = pre;
		}
		else {
			p->ltag = 0;
		}

		if (pre->rchild == NULL)
		{
			pre->rtag = 1;
			pre->rchild = p;
		}
		else {
			pre->rtag = 0;
		}
		pre = p;
		Thread(p->rchild);
	}
}

TBTree* CreatThread(TBTree* b)// 建立中序线索二叉树
{
	TBTree* root;
	root = (TBTree*)malloc(sizeof(TBTree));
	root->ltag = 0;
	root->rtag = 1;
	if (b == NULL)
		root->lchild = root;
	else {
		root->lchild = b;
		pre = root;
		Thread(b);
		pre->rchild = root;
		pre->rtag = 1;
		root->rchild = pre;
	}
	return root;
}

访问线索二叉树

1. 在中序线索二叉树上查找任意节点的中序前驱节点

//在中序线索二叉树上查找任意节点p的中序前驱节点
TBTree* Inpre(TBTree* p)
{
	TBTree* tmp;
	if (p->ltag == 1)
		return p->lchild;
	else {  // 有右孩子	:p的前驱节点就是以p为根节点的子树的最右节点
		TBTree* tmp = p->lchild;
		while (tmp->rtag == 0) //rtag为0表示有孩子
			tmp = tmp->rchild;
	}
}

2. 在中序线索二叉树上查找任意节点的中序后继节点

//在中序线索二叉树上查找任意节点的中序后继节点
TBTree* InPoost(TBTree* p)
{
	TBTree* tmp;
	if (p->rtag == 1) // 没有左孩子
		return p->rchild;
	else { // 有右孩子：则p的后继节点就是以p的右孩子为子树的最左节点
		tmp = p->rchild;
		while (tmp->ltag == 0)
			tmp = tmp->lchild;
	}
}

3. 中序遍历中序线索二叉树

void Inorder(TBTree* b)
{
	TBTree* p = b->lchild;
	while (p != b)
	{
		while (p->ltag == 0)  // 找到第一个节点
			p = p->lchild;
		printf("%c ",p->data); 

		while (p->rtag == 1 && p != b) // 当后继线索存在且不是头结点时
		{
			printf("%c ",p->data);
			p = p->rchild;
		}
		p = p->rchild;

	}
}

哈夫曼树(最优二叉树)

哈夫曼树构造

哈夫曼算法

树和森林

树的存储结构

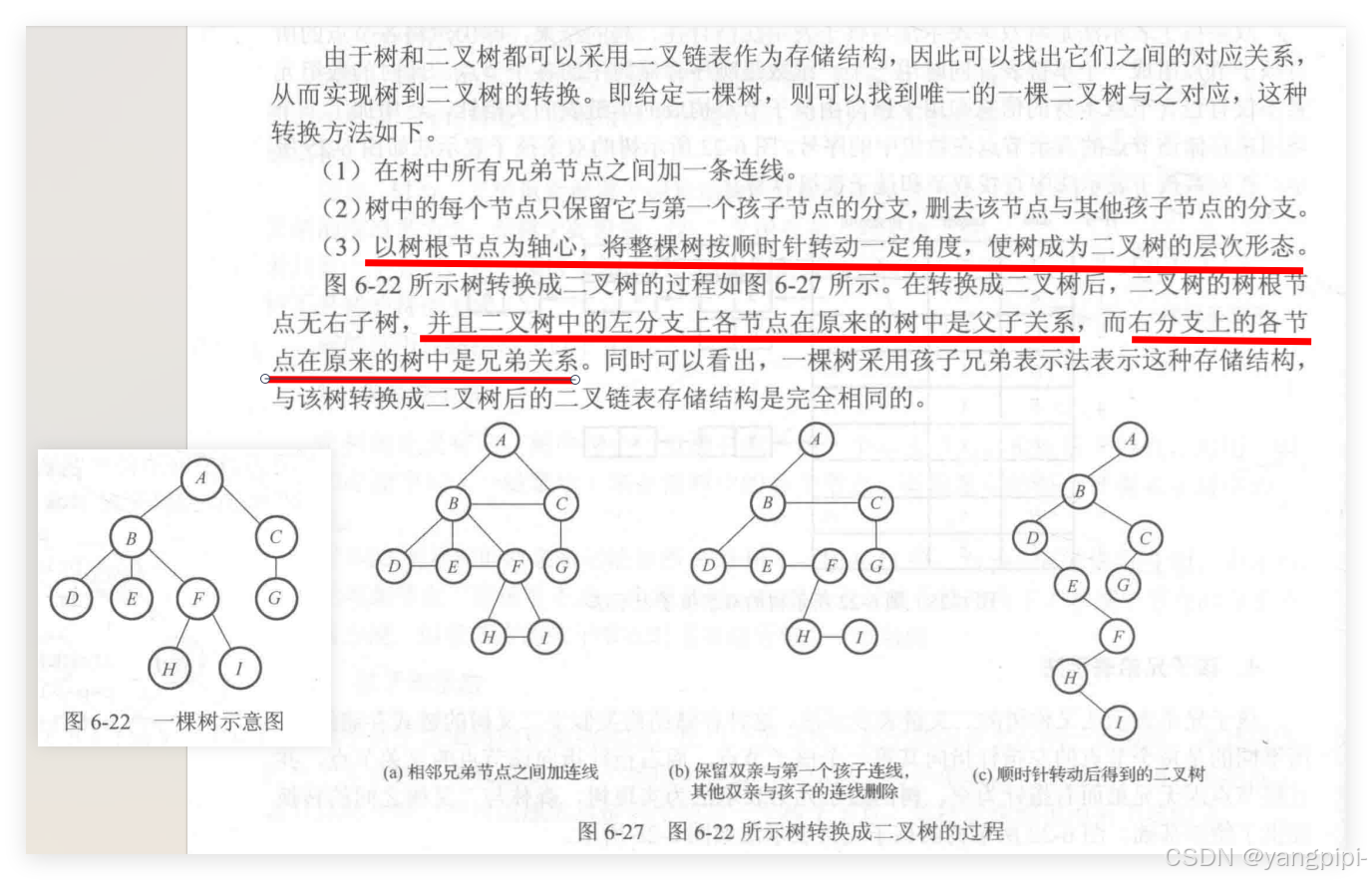
树->二叉树

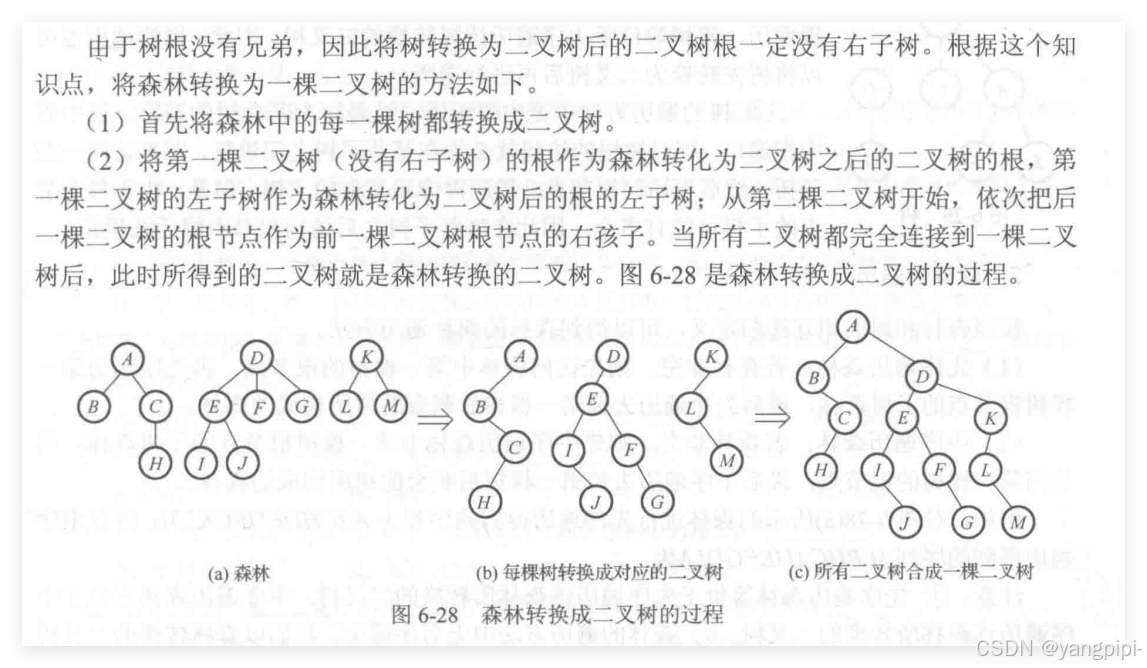
森林->二叉树

二叉树转换为树和森林

树的遍历

森林的遍历