算法第26天 | 贪心算法、455.分发饼干、376. 摆动序列、 53. 最大子序和

烨然若神人~2025-05-23 21:30

弹性算法理论基础

想清楚 局部最优 是什么，如果可以推导出全局最优，那就是正确的贪心算法

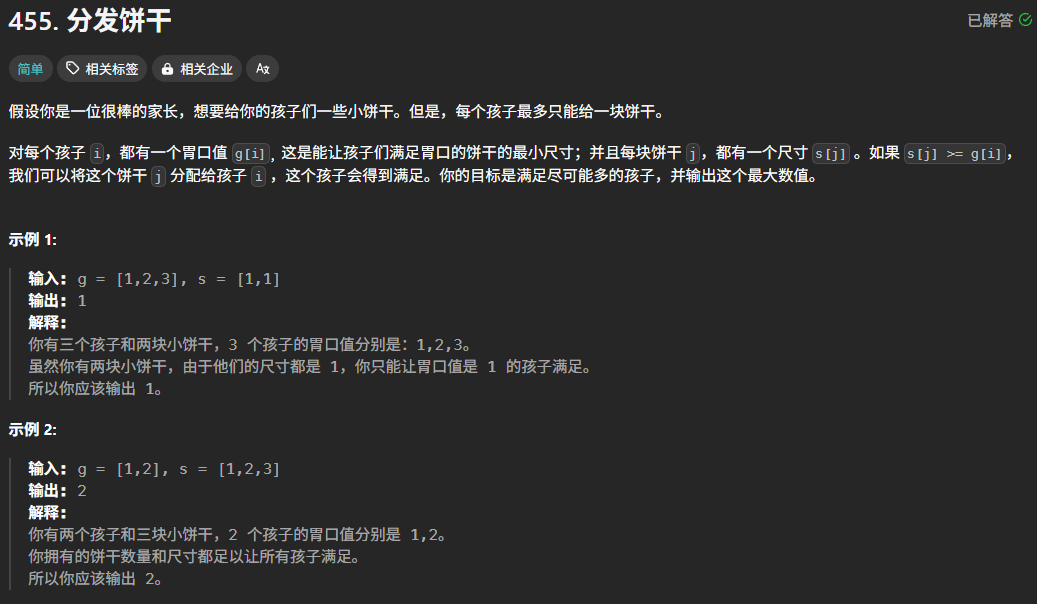
455. 分发饼干

题目

思路与解法

python 复制代码

class Solution:
    def findContentChildren(self, g: List[int], s: List[int]) -> int:
        res = 0
        i = 0
        j = 0
        g.sort()
        s.sort()
        while i < len(s) and j < len(g):
            if s[i] >= g[j]:
                res += 1
                j += 1
            i += 1
        return res

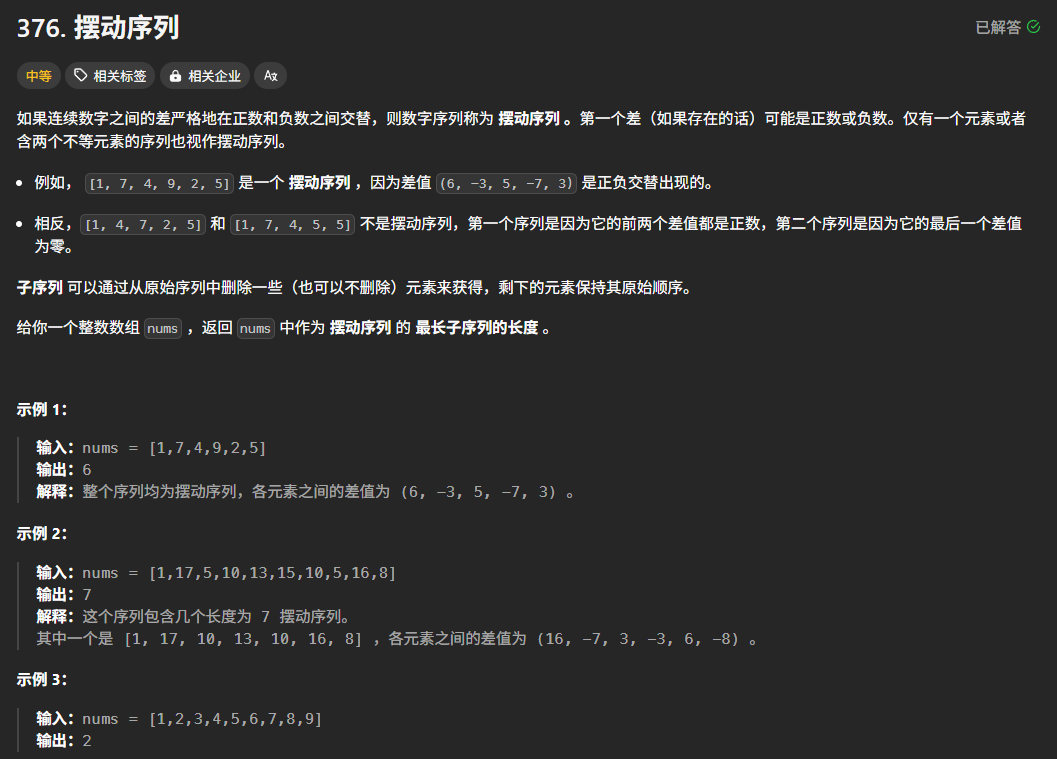
376. 摆动序列

题目

思路与解法

没太懂，但是不想细想了

python 复制代码

class Solution:
    def wiggleMaxLength(self, nums: List[int]) -> int:
        if len(nums) <= 1:
            return len(nums)  # 如果数组长度为0或1，则返回数组长度
        curDiff = 0  # 当前一对元素的差值
        preDiff = 0  # 前一对元素的差值
        result = 1  # 记录峰值的个数，初始为1（默认最右边的元素被视为峰值）
        for i in range(len(nums) - 1):
            curDiff = nums[i + 1] - nums[i]  # 计算下一个元素与当前元素的差值
            # 如果遇到一个峰值
            if (preDiff <= 0 and curDiff > 0) or (preDiff >= 0 and curDiff < 0):
                result += 1  # 峰值个数加1
                preDiff = curDiff  # 注意这里，只在摆动变化的时候更新preDiff
        return result  # 返回最长摆动子序列的长度

53. 最大子序和

题目

思路与解法

python 复制代码

class Solution:
    def maxSubArray(self, nums: List[int]) -> int:
        res = float('-inf')
        count = 0

        for i in range(len(nums)):
            count += nums[i]
            if count > res:
                res = count
            if count < 0:
                count = 0

        return res